1. Introduction
The physics of high-temperature superconductors have been studied intensively for more than 35 years since the discovery of high-temperature superconductivity [
1]. It is still a challenging issue to clarify the mechanism of high-temperature superconductivity. Since the parent materials of high-temperature cuprates are Mott insulators when no carriers are doped, high-temperature cuprates are typical strongly correlated electron systems. The strong correlation makes it hard to elucidate the mechanism of superconductivity. Thus, it is important to understand the electronic properties of strongly correlated electron systems.
The CuO
2 plane is commonly contained in various high temperature cuprates and consists of oxygen atoms and copper atoms. It is certain that the CuO
2 plane plays an important role in the emergence of high-temperature superconductivity [
2,
3,
4,
5,
6,
7,
8]. The fundamental and important model on this plane is the three-band d-p model [
4,
5,
6,
7,
8,
9,
10,
11,
12,
13,
14,
15,
16,
17,
18,
19,
20,
21,
22,
23,
24,
25,
26]. The two-dimensional (2D) Hubbard model is regarded as an effective model where we consider only
d electrons by integrating out the freedom of
p electrons. The 2D Hubbard model [
27,
28,
29] is also the basic model for cuprate superconductors.
The 2D Hubbard model contains fruitful physics although it looks very simple, and it may include effective interactions that induce electron pairing to bring about high-temperature superconductivity. The Hubbard model has been studied intensively to clarify the pairing mechanism of high-temperature superconductivity [
30,
31,
32,
33,
34,
35,
36,
37,
38,
39,
40,
41,
42,
43,
44,
45,
46,
47,
48,
49]. One may wonder why the effective attraction arises between electrons from the on-site repulsive Coulomb interaction. This effective pairing interaction may originate from the effective nearest-neighbor exchange coupling and the kinetic energy effect. On this subject, the ladder Hubbard model (two-chain model) has also been studied [
50,
51,
52,
53,
54,
55].
The Hubbard model was first introduced to understand the metal-insulator transition [
27]. Recent studies indicate the possibility of the existence of a superconducting (SC) phase in the parameter space of the hole density, the strength of Coulomb interaction
U and the next nearest-neighbor transfer integral $$\boldsymbol{t}^{\prime}$$ in the ground state [
47]. These three parameters are important and give plentiful structures of the phase diagram that include the superconducting phase and the antiferromagnetic phase. The transfer $$\boldsymbol{t}^{\prime}$$ plays an essential role in determining the stability of magnetic states. For example, in the case where $$\boldsymbol{t}^{\prime}=\boldsymbol{0}$$, the antiferromagnetic state becomes unstable when holes are doped. The 2D Hubbard model is also useful to understand the appearance of inhomogeneous electronic states such as stripes [
56,
57,
58,
59,
60,
61,
62,
63,
64,
65,
66,
67,
68,
69,
70,
71] and checkerboard-like density of states [
72,
73,
74,
75]; the existence of these inhomogeneous states has indeed been reported for high-temperature cuprates.
In the study of cuprate superconductors and also iron-based superconductors, lattice and charge effects play an important role. Inhomogeneous striped states could be stabilized associated with lattice distortions [
62]. Many interesting properties have been reported concerning lattice effects such as an anomalous isotope effect [
76,
77,
78,
79] and a shape resonance in a superlattice of quantum strings [
80,
81]. In the study of cuprate superconductors Bi
2Sr
2CaCuO
8+y and La
2CuO
4+y for which mobile oxygen interstitials by using local probes, a scenario has been shown that a strongly correlated Fermi liquid coexists with stripes that are made of anisotropic polarons condensed into a generalized Wigner charge density wave [
82,
83,
84].
The relation between the Hubbard model and the d-p model was investigated in the early state of the study of high-temperature cuprates by Feiner et al. [
85] They were able to reduce the d-p model into an effective one-band model by means of the cell-perturbation method. It has also been shown by numerical calculations that the Hubbard model and the three-band d-p model exhibit similar electronic properties [
14,
26].
In order to explore the superconducting ground state, it is favorable to suppress magnetic correlations and magnetic instabilities. For this purpose, we consider the strongly correlated region with large
U. The strong antiferromagnetic correlation is suppressed by doped hole carriers when
U is large. In this region we calculated superconducting properties in the 2D Hubbard model, and the existence of a superconducting phase is followed.
In Section 2, we discuss the critical temperature of superconductivity in many-electron systems. We discuss improved many-body wave functions in Section 3. In Section 4, we show the results obtained by the optimization variational Monte Carlo method. We show the SC order parameter as a function of
U and phase diagrams when we vary the hole density
x. We discuss the kinetic energy driven superconductivity in the strongly correlated region. We also examine the possibility of superconductivity in the nematic charge-ordered phase. In Section 5, we exhibit pair correlation function as a function of lattice sites. This shows that the pair correlation function is almost constant at long distances and the wave function indeed has long-range superconducting order in the strongly correlated region. We also discuss the duality of strong electron correlation, which means that the strong correlation can be an origin of attractive interaction of
d-wave electron pairs and at the same time, it suppresses the pair correlation function.
2. Superconductivity in Many-Electron Systems
It is reasonable to expect that when the energy scale of an interaction is very large, we can expect superconductivity with high critical temperature
Tc. Since the energy scale of the Coulomb interaction is of the order of eV, the Coulomb interaction is one of the candidates to give high-temperature superconductivity. For materials shown in , we can confirm that the following empirical relations hold for the superconducting critical temperature:
where
t denotes the transfer integral, and
m∗ and
m0 are the effective mass and bare mass of electrons, respectively. The shows typical values of
t, the ratio
m∗⁄
m0 and
Tc. The order of
Tc for correlated electron materials is consistent with the formula in Equation (1). For high-temperature cuprates, the transfer integral
t is estimated as
t ~ 0.51 eV and
TC is of the order of 100 K. Since the transfer
t of iron pnictides is about five times smaller than that of cuprates, iron pnictides have lower
Tc than cuprate superconductors. The critical temperature
Tc of heavy fermions is very low although heavy fermion materials are strongly correlated electron systems. This is due to large effective mass of
f electrons which is as large as 100~1000 times the band (bare) mass
m0. Then the characteristic energy scale is reduced considerably so that
Tc is of the order of 1 K.
. The transfer integral t, effective mass ratio m∗⁄m0 and critical temperature Tc in correlated electron systems. For Hydrides, the Debye frequency ωln is shown instead of t. For heavy fermion materials, t⁄(m∗⁄m0) corresponds to the Kondo temperature TK.
3. Optimization Variational Monte Carlo Method
3.1. Hamiltonian
We consider the two-dimensional Hubbard model that is one of simplest model in correlated electron systems. The Hamiltonian is given by
where
tij indicates the transfer integral which takes the value $$t_{ij}=-t$$ when
i and
j are nearest-neighbor pairs and $$t_{ij}=-t^{\prime}$$ when
i and
j are next nearest-neighbor pairs.
U denotes the strength of the on-site repulsive Coulomb interaction. The energy is measured in units of
t throughout this paper.
3.2. Many-Body Wave Functions
3.2.1. Optimized Many-Body Wave Functions
The wave function of non-interacting many fermions is written as a Slater determinant. In a weakly interacting many-fermion system, the wave function shows a deviation from the simple Slater determinant. In many-fermion systems with strong interaction between fermions, we should consider strong correlation in many-body wave functions. For the Hubbard Hamiltonian with large interaction
U, one convincing way to construct the many-fermion wave function is to start from the Gutzwiller wave function. The Gutzwiller wave function is written as
where
ψ0 is one-particle state given by a Slater determinant and
PG denotes the Gutzwiller operator that is given as
where
g is the variational parameter in the range of 0 ≤
g ≤ 1. We usually take
ψ0 as the Fermi sea, the BCS wave function or a state with some magnetic or charge orders.
The Gutzwiller wave function can be improved by several ways. One is the well-known Jastrow function; this is written as
where the Jastrow operator
PJ is given by
where
dj is the operator for the doubly occupied site (so called doublon operator) given by $$d_j=n_{j\uparrow}n_{j\downarrow}$$, and
ej is that for the empty site (holon operator) given as $$e_j=(1-n_{j\uparrow})(1-n_{j\downarrow})$$.
τ runs over all nearest-neighbor sites
j.
η is introduced as the variational parameter in the range of 0 ≤
η ≤ 1.
The other effective way to improve the wave function is to multiply by the exponential operator
e−λK [
46,
47,
48,
92,
93,
94,
95,
96,
97]:
where
K is the non-interacting part of the Hamiltonian, which is called the kinetic operator in this paper, and is given by
The variational parameter
λ is introduced to minimize the expectation value of the ground-state energy. This wave function can be improved further by multiplying by the Gutwiller operator and the kinetic operator again [
46,
93]:
where $$P_G(g^{\prime})$$ is the Gutzwiller operator with variational parameter $$g^{\prime}$$. $$λ^{\prime}$$ and $$g^{\prime}$$ are in general different from
λ and
g, respectively. We have correlated wave functions $$\psi_G,\, \psi_\lambda^{(1)}\equiv\psi_\lambda, \psi_\lambda^{(2)}, \psi_\lambda^{(3)}$$, and it is possible to generalize further.
We discuss the stability of superconducting state and magnetically ordered states by using this kind of improved and optimized wave functions. We can also discuss the metal-insulator transition on the basis of this wave function where the strong correlation between electrons plays an essential role [
97].
3.2.2. Correlated Superconducting Wave Function
The correlated superconducting state is formulated starting from the BCS wave function. The BCS wave function is written as
The coefficients
uk and
vk appear in the ratio $$u_{k}/v_{k}=\Delta_{k}/\left(\xi_{k}+\sqrt{\xi_{k}^{2}+\Delta_{k}^{2}}\right)$$ with the gap function ∆
k and $$\xi_k=\epsilon_k-\mu $$ where
μ is the chemical potential. For the d-wave paring, we take $$\Delta_{k}=\Delta_{s}\big(\cos k_{x}-\cos k_{y}\big)$$. We usually first consider the BCS state with the Gutzwiller operator given by
where $$P_{N_{e}}$$ stands for the operator that extracts the state with
Ne electrons. This wave function was referred to as the resonating valence bond state (RVB) by Anderson [
98].
In our formulation the correlated superconducting wave function is given as
In this wave function the operator $$P_{N_{e}}$$ is not used because of the numerical method to evaluate expectation values, while in the Gutzwiller BCS state $$\psi_{G-BCS}$$, the total number of electrons is fixed. Because we use the auxiliary filed method in a Monte Carlo simulation [
46,
99], we perform the electron-hole transformation for down-spin electrons: $$d_{k}=c_{-k\downarrow}^{\dagger}, d_{k}^{\dagger}=c_{-k\downarrow}$$, and the operator for up-spin electrons remains the same [
93]. We put $$c_k=c_{k\uparrow}$$ and $$c_k^\dagger=c_{k\uparrow}^\dagger $$. The electron-pair operator $$c_{k\uparrow}^\dagger c_{-k\downarrow}^\dagger $$ is transformed to the mixing operator $$c_k^\dagger d_k$$. This transformation indicates that
ci =
ci↑ and $$d_i=c_{i\downarrow}^\dagger $$ in the real space representation. In the real space, the
d-wave anisotropic pairing order parameters are assigned to each bond between the site
i and its nearest-neighbor sites $$i+\hat{x}$$ and $$i+\widehat{y}$$ where $$\hat{x}$$ and $$\hat{y}$$ denote the unit vectors in the $$\hat{x}$$ and $$\hat{y}$$ directions, respectively. We assign the following order parameter in the real space representation:
3.2.3. $$e^{-\lambda K}$$ and the Renormalization of High-Energy Excitations
Let us discuss the role of $$e^{-\lambda K}$$ introduced in improved wave functions. It is easily seen that the operator $$e^{-\lambda K}$$ suppresses the weight of high-energy excitation modes because $$e^{-\lambda K}$$ becomes small for high-energy states. Thus $$e^{-\lambda K}$$ plays a role like the projection operator that projects out low-lying excitation modes. This means that the role of $$e^{-\lambda K}$$ is analogous to that of the renormalization group procedure, where the cutoff Λ is reduced to Λ −
dΛ, the states near the Fermi surface are magnified and their contributions increase [
100]. The parameter
λ controls contributions from high-energy modes, which magnifies the states near the Fermi surface.
4. Phase Diagram by the Optimization Variational Monte Carlo Method
4.1. Superconductivity and Antiferromagnetic State
In this section, we discuss possible phases of the 2D Hubbard model including superconducting and antiferromagnetic states when we vary the strength of the Coulomb interaction
U. First, we show the result obtained by using the BCS-Gutzwiller wave function. The ground-state energy has a minimum at finite Δ
s for the BCS-Gutzwiller function with
d-wave symmetry in the 2D Hubbard model [
35,
36]. The SC condensation energy
Econd per site was evaluated in the limit of large system size
N → ∞ (where
N is the number of sites). We obtained in this limit
Here we set
t = 0.5 eV. We obtained a similar result for the three-band d-p model [
19]. This indicates that the SC condensation energy per atom is approximately given by 0.2 meV which is of the order of 10
−4 eV. In experiments, the condensation energy was estimated based on the result of specific heat measurements for YBCO [
35,
101]. The result is
per Cu atom. We obtain the similar value of the condensation energy from the data of the critical magnetic field [
102]. Hence, we have a remarkable agreement between theoretical evaluations and experimental measurements. We can say that the characteristic energy scale of cuprate high-temperature superconductors is given by this value.
We turn to the results obtained by the improved wave function
ψλ. We show the antiferromagnetic and superconducting order parameters as a function of
U/t in where calculations were carried out for the 2D Hubbard model on a 10 × 10 lattice with $$t^{\prime}=0$$ and
Ne = 88. The characteristic feature of the 2D Hubbard model is that the antiferromagnetic (AF) correlation is strong and the AF state is easily stabilized when
U is moderately large. We also have the SC phase when
U is as large as the bandwidth or larger than it. When $$t^{\prime}=0$$, the AF correlation weakens upon carrier doping, and it vanishes when
U is very large around
U/t ≃ 18 for the hole density
x = 0.12. The SC phase can exist as a pure
d-wave state when
U/t is about 18.
. Antiferromagnetic and superconducting order parameters as a function of
U/t where $$t^{\prime}=0$$ and
Ne = 88 for the 2D Hubbard model on a 10 × 10 lattice (figure from [
47] with a slight modification). Δ indicates the AF order parameter Δ
AF or the SC order parameter Δ
s. We impose the periodic boundary condition in one direction and antiperiodic boundary condition in the other direction. AF(G) indicates the result obtained by using the Gutzwiller function. The results AF and SC show those for the improved wave function
ψλ.
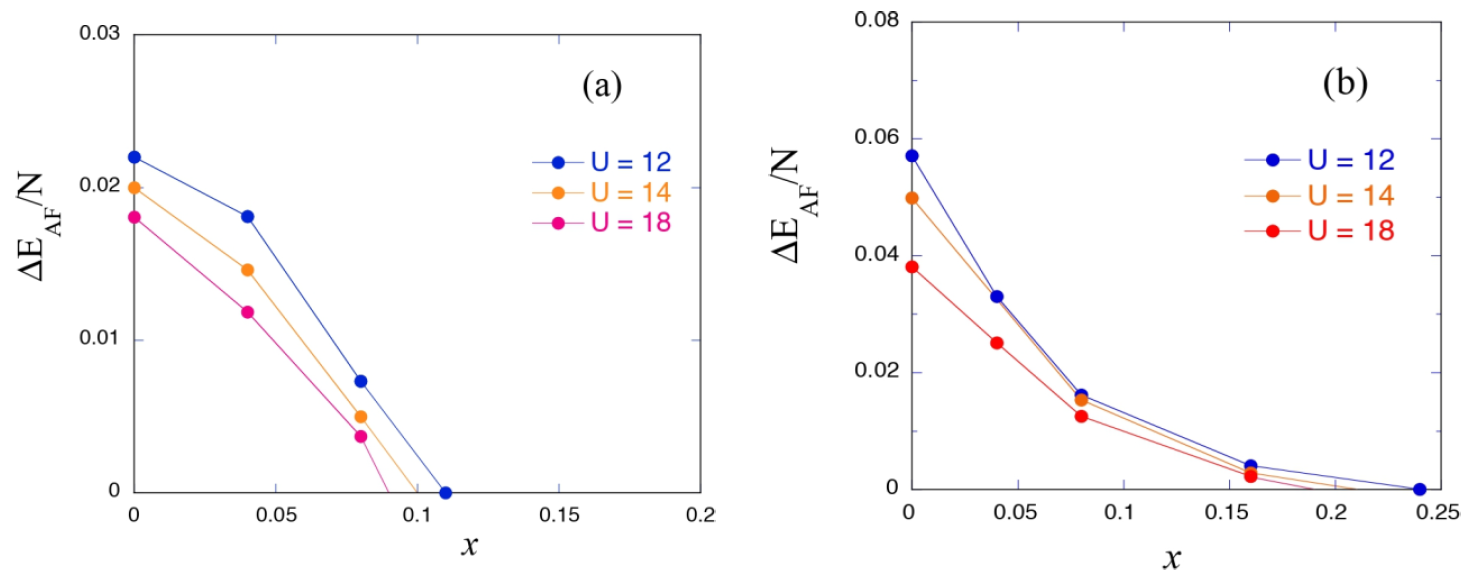
The next nearest-neighbor transfer $$t^{\prime}$$ plays a significant role concerning the stability of the AF state. In , we show the AF condensation energy as a function of the hole doping rate x for the 2D Hubbard model on a 10 × 10 lattice. The AF condensation energy is defined as $$\Delta E_{AF}=E(\Delta_{AF}=0)-E(\Delta_{AF,\mathrm{opt}})$$ where ∆
AF is the AF order parameter and ∆
AF,opt is its optimized value. In the case of vanishing $$t^{\prime}$$, ∆
EAF vanishes at
x = 0.1 when
U is greater than 14
t (a), while ∆
EAF remains finite (positive value) even for large
U and large carrier density when $$t^{\prime}$$ = −0.2 (b). The instability of the AF state for $$t^{\prime}$$ = 0 is closely related to the kinetic energy of electrons (holes). Since the kinetic energy gain in the AF state is suppressed as
U increases, the total energy lowering due to the AF ordering and kinetic energy gain will get smaller for large
U. Then, in order to lower the ground-state energy, the AF order will be suppressed to increase the kinetic energy gain. Finally, the AF order disappears when
U becomes as large as the critical value. This is the mechanism of vanishing AF order in the strongly correlated region.
. The AF condensation energy ∆
EAF per site as a function of the doping rate
x for several values of
U/t (where
U/t = 12, 14 and 18) on a 10 × 10 lattice. We put (
a) $$t^{\prime}=0$$ and (
b) $$t'=-0.2t$$ [
48].
We consider the SC condensation energy defined by $$\Delta E_{SC}=E(\Delta_{s}=0)-E\big(\Delta_{s,\mathrm{opt}}\big)$$ where ∆
s,opt is the optimized value of ∆
s to give the lowest ground-state energy. In , ∆
ESC and ∆
EAF are shown as a function of the doping rate
x for
U/t = 18 and $$t^{\prime}=0$$ on a 10 × 10 lattice. In the low doping region, there is the AF insulating (AFI) phase for 0 ≤
x ≲ 0.06. The AFI is an insulating phase because of an instability toward the phase separation where the charge susceptibility
χc becomes negative.
χc is defined as
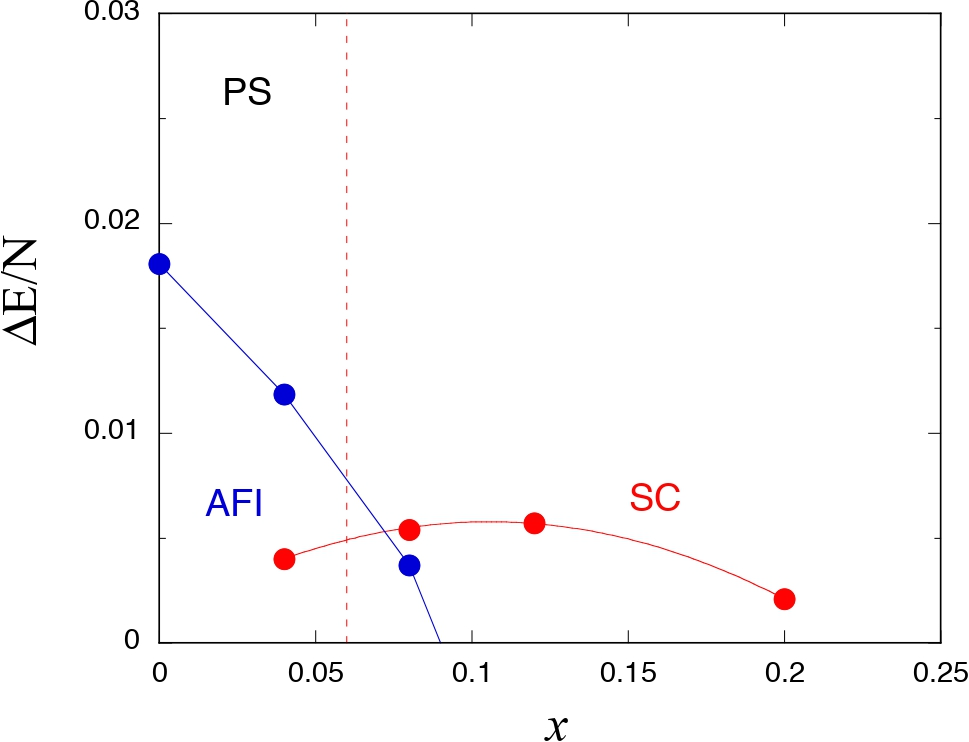
where
E(
Ne) is the ground-state energy when the number of electrons is
Ne. The negative sign of
χc indicates that the ground state is an insulator. The SC condensation energy ∆
ESC is finite for 0.05 ≲
x ≲ 0.2. There is a coexistent metallic phase of SC and AF when 0.06 ≲
x ≲ 0.09. The pure
d-wave SC phase is in the range 0.09 ≲
x ≲ 0.2. The typical energy scale of SC state is given by ∆
ESC~0.005
t and ∆
s~0.01
t. The corresponding AF values are much larger than those of SC values. It has been shown that ∆
EAF is reduced when we improve the wave function from
ψλ = $$\psi_{\lambda}^{(1)}\mathrm{~to~}\psi_{\lambda}^{(3)}$$ [
48]. We here mention that the existence of AFI phase would depend on the value of $$t^{\prime}$$. When $$t^{\prime}$$ is negative, the AFI phase will disappear as |$$t^{\prime}$$| increases.
. The condensation energy per site as a function of the hole doping rate
x for the 2D Hubbard model on a 10 × 10 lattice (figure from [
48] with a slight modification). The AF and SC condensation energies are shown. We set $$t^{\prime}=0$$ and
U/t = 18. AFI indicates the AF insulating phase and SC shows the
d-wave SC phase. At about
x ≃ 0.06, the AF state changes from an insulator to a metallic state as
x increases. We have the coexistent state of antiferromagnetism and superconductivity for 0.06 ≲
x ≲ 0.09.
In strongly correlated electron systems, the kinetic energy effect is important in determining the stable ground state. The kinetic energy effect in superconductivity has been examined for electronic models [
103,
104,
105,
106,
107,
108,
109,
110,
111,
112]. We discuss the role of the kinetic term in this subsection. For this purpose, we define two contributions to ∆
ESC from the kinetic term and the potential term, respectively:
where
Ekin and
EU are expectation values of the kinetic term
K and the Coulomb term $$U\sum\nolimits_in_{i\uparrow}n_{i\downarrow}$$, respectively. From the definition we have
In the BCS theory, the attractive interaction brings about superconductivity, and thus the interaction term
V gives the SC condensation energy, that is,
V in the SC state is lower than that in the normal state:
δV < 0 (the variation of
V is negative when the interaction is introduced).
V will give the positive contribution to Δ
ESC This is also the case for weak coupling superconductivity. In fact, for the Gutzwiller-BCS wave function in the moderately correlated region, we have
Instead, in the strongly correlated region where
U is as large as 18
t, we obtain for
ψλ as
The kinetic part gives a positive contribution to ∆
ESC. We also define
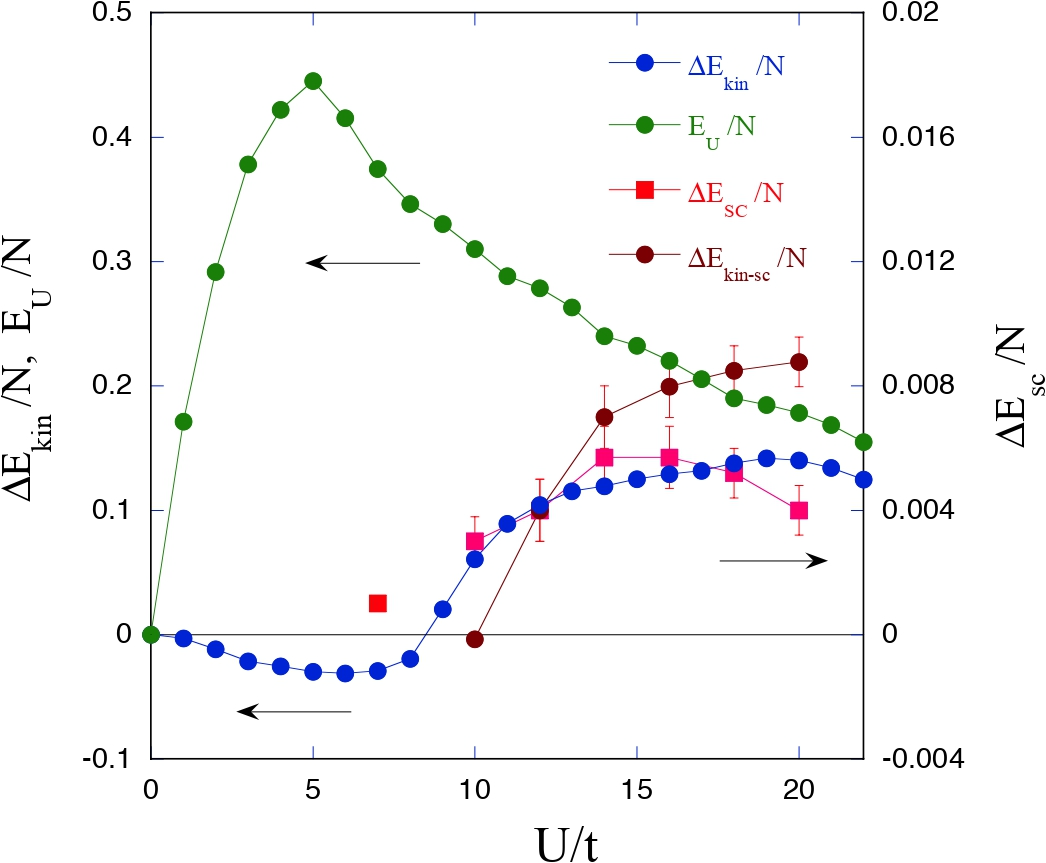
where
Ekin (
ψG) and
Ekin (
ψλ) are kinetic energies for
ψG and
ψλ, respectively. We show ∆
Ekin, $$\Delta E_{kin-sc}$$ and ∆
ESC as well as
EU (the expectation value of the interaction term) in . In we put
x = 0.12 and $$t^{\prime}$$ = 0. The shows that ∆
Ekin changes its sign and begins to increase as
U increases when
U ≳ 8
t. $$\Delta E_{kin-sc}$$ becomes positive in the strongly correlated region and shows a similar behavior to ∆
Ekin. This behavior is consistent with the analysis for Bi
2Sr
2CaCu
2O
8+d [
103].
. The kinetic-energy difference (∆
Ekin) ⁄
N, the Coulomb energy
EU ⁄N(left axis), the kinetic-energy gain ∆
Ekin-sc/
N and the SC condensation energy ∆
ESC ⁄N (right axis) as a function of
U/t on a 10 × 10 lattice where
Ne = 88 and $$t^{\prime}=0$$ [
102]. We use the periodic boundary condition in one direction and antiperiodic boundary condition in the other direction. The vertical axis on the right side shows the SC condensation energy ∆
ESC ⁄
N and the kinetic condensation energy ∆
Ekin-sc ⁄
N.
The existence of striped states has been pointed out by many authors in cuprate superconductors and in the 2D Hubbard model [
43,
56,
57,
58,
59,
60,
61,
62,
63,
64,
65,
66,
67,
68,
69,
70,
71]. We do not take into account the lattice effect here, although it helps the formation of charge-ordered state,. The charge and spin modulations are described as
where
ρ and
m ≡ ∆
AF are variational parameters for charge and spin modulations, respectively.
r0 indicates the position of the domain boundary of spin modulation. For the commensurate AF state, we take
Qs = (
π,
π) and
ρ = 0. The stripe state is represented by the incommensurate wave vector
Qs = (
π ± 2
πδ,
π) where
δ stands for the incommensurability that is the inverse of the period of the AF order in the
x-direction. In this state, two adjacent AF magnetic domains are separated by a one-dimensional domain wall in the
y-direction. We have a
π-phase shift when crossing a domain wall. For the charge modulation, we put
Qc = 2
Qs so that the charge modulation period is just half of the spin modulation period.
We consider the region with the doping rate given by
x ⋍ 1/8. The stripe state is usually most stable in this region. We consider, however, the large-U case where the AF order disappears as described above. In this case we have the ground state with charge order and without magnetic order for which
m = ∆
AF = 0 and
ρ ≠ 0. This state is called the nematic state. The calculation was carried out for
U/t = 18, $$t^{\prime}$$ = 0 and
δ = 1/4 (4-lattice charge periodicity) with the electron number
Ne = 228 on a 16 × 16 lattice. The charge-ordered nematic state is indeed stabilized for this set of parameters. We examine how superconductivity exists in the charge-ordered state. Let us consider the following gap function:
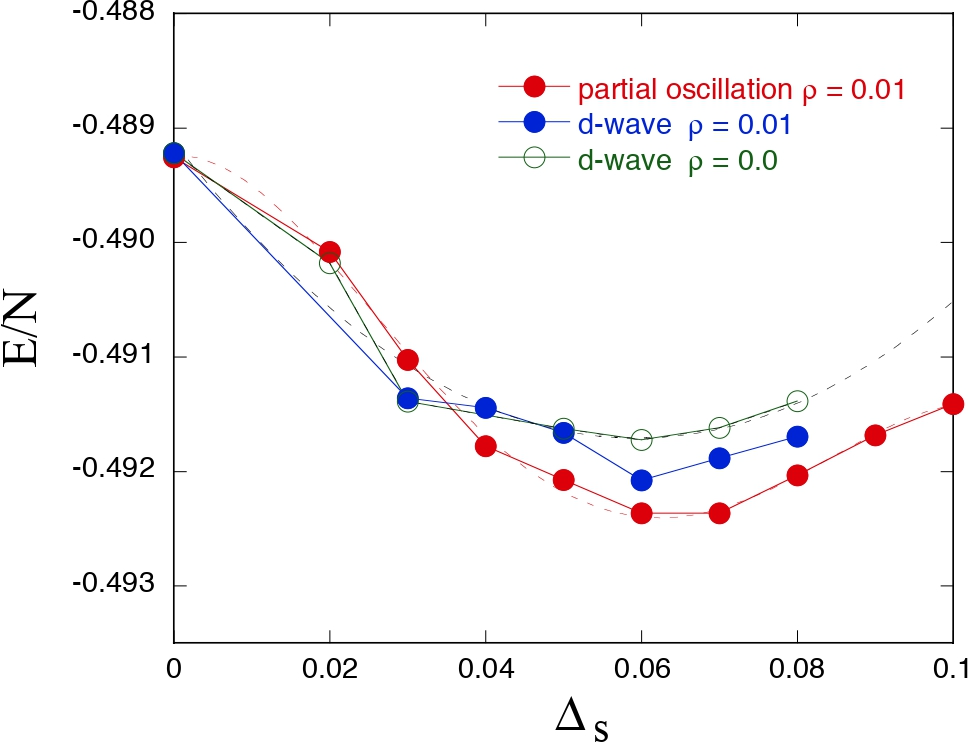
where the coordinate of site
i is
ri = (
x,
y) and
α is a real parameter. The hole (or electron) rich domains exist at
x = 4, 8, 12 and 16 for
α > 0 (or
α < 0). The gap function is spatially oscillating according to the charge modulation in this pairing state. In we show the ground-state energy per site
E⁄N as a function of ∆
s for the uniform
d-wave state and the oscillating d-wave state. The result shows that the oscillating
d-wave pairing state is most stable and will be realized. The superconducting state can coexist with inhomogeneous charge order with increased gap function. This gives a possibility that superconductivity is enhanced with higher
Tc in cooperation with the inhomogeneous nematic charge ordering.
. The ground-state energy per site as a function of the SC order parameter ∆s for U/t = 18 and $$t^{\prime}=0$$ at Ne = 228 on a 16 × 16 lattice. We used g = 0.005, λ = 0.055 and ρ = 0.01. We compare three energy expectation values for the wave function with uniform d-wave symmetry with ρ = 0 and 0.01, and that with partially oscillating d-wave pairing (α = −0.1). The dotted lines are guide for eyes.
5. Superconductivity and Strong Correlation
In this section, we examine the effect of strong correlation on superconductivity. We consider the effect of the Gutzwiller operator
PG on the superconducting correlation function. The BCS wave function
ψBCS (Δ
s) clearly shows the long-range correlation. In , we show the SC correlation function $$D_{sc}(\ell)\equiv\langle\Delta^\dagger(i)\Delta(i+\ell)\rangle $$, as a function of the lattice site for
Ne = 88,
U = 18
t and $$t^{\prime}=0$$ on a 10 × 10 lattice. Here the pair annihilation operator ∆(
i) at the site
i is defined by
where
for
α =
x and
y. $$\hat{\alpha}$$ stands for the unit vector in the
α-th direction.
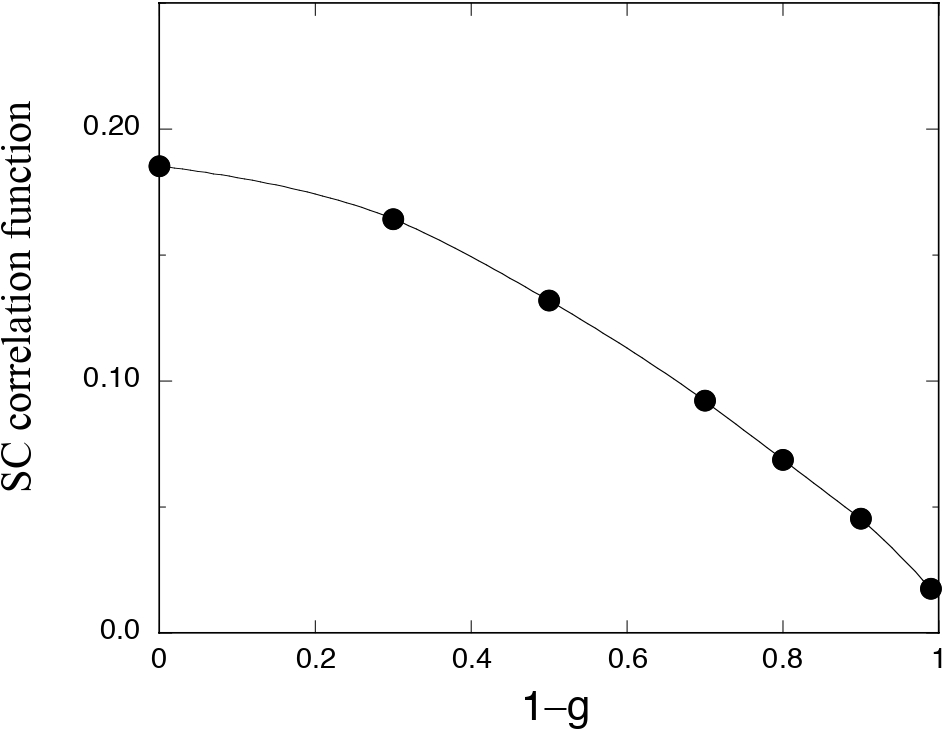
shows that the pair correlation function for
U = 18
t is almost constant when $$\ell$$ is large indicating that the ground state is superconducting. The values of $$D_{sc}(\ell)$$ for large $$\ell$$ are suppressed considerably compared to that for the non-interacting BCS wave function. This suppression is due to the strong correlation between electrons. This makes it rather hard to confirm the existence of the superconducting phase in numerical calculations of pair correlation functions by, for example, quantum Monte Carlo calculations. In , we show the SC correlation function $$D_{sc}(\ell)$$ of $$P_G\psi_{BCS}(\Delta_s)$$ at the site $$\ell=R_{max}=(5,5)$$ with
i = (1,1) as a function of 1 −
g for ∆
s = 0.05
t on a 10 × 10 lattice.
Rmax is the most distant point from the site
i = (1,1). indicates that the pair correlation function is suppressed by the electron correlation that is now given by the Gutzwiller on-site operator. Thus, we can say that the electron correlation has duality. This means that the electron correlation is an origin of attractive interaction between electrons and at the same time suppresses pair correlation functions.
The electron correlation has also an effect on the superconducting order parameter ∆. ∆ is defined by
We show ∆ as a function of 1 −
g in . ∆ exhibits a similar behavior to $$D_{sc}(\ell)$$, that is, ∆ is reduced by
PG.
. The pair correlation function $$D_{sc}(\ell)$$ for Ne = 88, U = 18t and $$t^{\prime}=0$$ on a 10 × 10 lattice where i = (1,1) and $$\ell$$ = (1,1),(1,2), (1,3), (1,4), (1,5), (2,5), (3,5), (4,5) and (5,5). The figure includes $$D_{sc}(\ell)$$ for U = 0 (squares), that for the BCS wave function $$\psi_{BCS}(\Delta_s)$$ with ∆s= 0.05t (open circles), and that for U = 18t (filled circles).
Figure 7. The pair correlation function $$D_{sc}(\ell)$$ for $$\ell=R_{max}=(5,5)$$ of $$P_G\psi_{BCS}(\Delta_s)$$ with ∆s = 0.05t on a 10 × 10 lattice. The parameter g is in the range of 0 ≤ g ≤ 1 and 1 − g = 0 corresponds to the BCS wave function.
When
g < 1. Hence the electron correlation also leads to the reduction of the SC gap ∆. The strong electron correlation has duality, which means that the electron correlation becomes an origin of attractive interaction of
d-wave pairing and at the same time, it suppresses SC correlation function and SC gap. One origin of this suppression is certainly the renormalization of the effective transfer integral and the effective mass. The heavy effective mass
m∗⁄
m reduces pair correlation functions and is not favorable for superconductivity as indicated by Equation (1). The exponential factor $$e^{-\lambda K}$$ could play a role in increasing pair correlation by the kinetic energy effect.
. The superconducting order parameter ∆ as a function of 1 − g for $$P_G\psi_{BCS}(\Delta_s)$$ with ∆s = 0.05t on a 10 × 10 lattice.
6. Discussion
The many-body wave function is important in the study of strongly correlated electron systems. We have constructed many-body wave functions starting from the Gutzwiller function to take into account strong correlation between electrons. The series $$\psi_{G},\psi_{\lambda}^{(1)}\equiv\psi_{\lambda},\psi_{\lambda}^{(2)},\psi_{\lambda}^{(3)},\cdots$$, will approach the exact wave function.
An instability toward magnetic ordering easily occurs in the two-dimensional Hubbard model. In particular, near the half-filled case with a small number of holes, the ground state has inevitably some magnetic or charge orders. Thus, we considered the strong correlated region where magnetic correlations and magnetic instabilities are suppressed.
Thus, we need a method of calculation by which we can evaluate physical properties in the strongly correlated region. This was the purpose of the study in this paper. We chose the value
U/t = 18 in this paper. Since the extreme strong correlation reduces the pair correlation function, it is favorable that we can choose a moderate value of U being less than
U = 18
t. We expect that this value is reduced when we take account of further improved wave functions $$\psi_{\lambda}^{(3)}$$, $$\psi_{\lambda}^{(4)}$$,⋯. In fact, the antiferromagnetic correlation is suppressed for the improved wave function $$\psi_{\lambda}^{(3)}$$[
48]. We expect that this will lead to a superconducting state with larger gap function.
7. Conclusions
We have investigated the correlated superconducting state in the ground state of the two-dimensional Hubbard model based on the optimization variational Monte Carlo method. First, we discussed that the SC condensation energy obtained by numerical calculations is consistent with that estimated from experimental results for high-temperature cuprate superconductors. Second, we presented the phase diagram as a function of U based on improved many-body wave functions. The superconducting phase exists in the strongly correlated region where U is larger than the bandwidth. When $$t^{\prime}=0$$, the AF correlation weakens upon hole doping in the strongly correlated region and the pure d-wave SC is realized. Third, we have also shown the phase diagram as a function of the carrier density x, where basically there are three phases: antiferromagnetic insulating phase, metallic antiferromagnetic phase and superconducting phase. Fourth, then we discussed the kinetic energy effect that would assist the appearance of superconductivity and this effect may play an important role in the realization of high-temperature superconductivity. Fifth, we investigated the cooperation of charge inhomogeneous order and superconductivity. This indicates the possibility that the superconducting critical temperature Tc will increase due to the coexistence with nematic charge ordering. Lastly, we showed the pair correlation function $$D_{sc}(\ell)$$. We discussed the effect of strong electron correlation on pair correlation function and SC order parameter. The pair correlation function is suppressed by the electron correlation operator PG. Although the correlation function $$D_{sc}(\ell)$$ becomes small due to PG, the long-range order still exists for ψλ.
Acknowledgments
The author expresses his sincere thanks to K. Yamaji and M. Miyazaki for fruitful discussions. This work was supported by a Grant-in-Aid for Scientific Research from the Ministry of Education, Culture, Sports, Science and Technology of Japan (Grant No. 17K05559). A part of computations was supported by the Supercomputer Center of the Institute for Solid State Physics, the University of Tokyo. The numerical calculations were also carried out on Yukawa-21 at the Yukawa Institute for Theoretical Physics in Kyoto University.
Author Contributions
Formal Analysis, Investigation, Resources, Writing—Original Draft Preparation, T.Y.
Ethics Statement
Not applicable.
Informed Consent Statement
Not applicable.
Funding
Grant-in-Aid for Scientific Research from the Ministry of Education, Culture, Sports, Science and Technology of Japan (Grant No. 17K05559).
Declaration of Competing Interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
References
1.
Bednorz JB, Müller KA. Possible high
Tc superconductivity in the Ba−La−Cu−O system.
Z. Phys. B 1986,
64, 189–193.
[Google Scholar]
2.
McElroy K, Simmonds RW, Hoffman JE, Lee D-H, Orenstein J, Eisaki H, et al. Relating atomic-scale electronic phenomena to wave-like quasiparticle states in superconducting Bi
2Sr
2CaCu
2O
8+δ.
Nature 2003,
422, 592–596.
[Google Scholar]
3.
Hussy NE, Abdel-Jawad M, Carrington A, Mackenzie AP, Balicas L. A coherent three-dimensional Fermi surface in a high-transition-temperature superconductor.
Nature 2003,
425, 814–817.
[Google Scholar]
4.
Weber C, Haule K, Kotliar G. Optical weights and waterfalls in doped charge-transfer insulators: A local density approximation and dynamical mean-field theory study of La
2−xSr
xCuO
4.
Phys. Rev. B 2008,
78, 134519.
[Google Scholar]
5.
Hybertsen MS, Schluter M, Christensen NE. Calculation of Coulomb-interaction parameters for La2CuO4 using a constrained-density-functional approach.
Phys. Rev. B 1989,
39, 9028.
[Google Scholar]
6.
Eskes H, Sawatzky GA, Feiner LF. Effective transfer for singlets formed by hole doping in the high-
Tc superconductors.
Physica C 1989,
160, 424–430.
[Google Scholar]
7.
McMahan AK, Annett JF, Martin RM. Cuprate parameters from numerical Wannier functions.
Phys. Rev. B 1990,
42, 6268.
[Google Scholar]
8.
Eskes H, Sawatzky G. Doping dependence of high-energy spectral weights for the high-
Tc cuprates.
Phys. Rev. B 1991,
43, 119.
[Google Scholar]
9.
Emery VJ. Theory of high-
Tc superconductivity in oxides.
Phys. Rev. Lett. 1987,
58, 2794.
[Google Scholar]
10.
Hirsch JE, Loh EY, Scalapino DJ, Tang S. Pairing interaction in CuO clusters.
Phys. Rev. B 1989,
39, 243.
[Google Scholar]
11.
Scalettar RT, Scalapino DJ, Sugar RL, White SR. Antiferromagnetic, charge-transfer, and pairing correlations in the three-band Hubbard model.
Phys. Rev. B 1991,
44, 770.
[Google Scholar]
12.
Oguri A, Asahata T, Maekawa S. Gutzwiller wave function in the three-band Hubbard model: A variational Monte Carlo study.
Phys. Rev. B 1994,
49, 6880.
[Google Scholar]
13.
Koikegami S, Yamada K. Antiferromagnetic and Superconducting Correlations on the d-p Model. Journal of the Physical Society of Japan.
J. Phys. Soc. Jpn. 2000,
69, 768–776.
[Google Scholar]
14.
Yanagisawa T, Koike S, Yamaji K. Ground state of the three-band Hubbard model.
Phys. Rev. B 2001,
64, 184509.
[Google Scholar]
15.
Koikegami S, Yanagisawa T. Superconducting gap of the two-dimensional d-p model with small
Ud.
J. Phys. Soc. Jpn. 2001,
70, 3499–3502.
[Google Scholar]
16.
Yanagisawa T, Koike S, Yamaji K. Lattice distortions, incommensurability, and stripes in the electronic model for high-
Tc cuprates.
Phys. Rev. B 2003,
67, 132408.
[Google Scholar]
17.
Koikegami S, Yanagisawa T. Superconductivity in Sr
2RuO
4 mediated by Coulomb scattering.
Phys. Rev. B 2003,
67, 134517.
[Google Scholar]
18.
Koikegami S, Yanagisawa T. Superconductivity in multilayer perovskite: Weak coupling analysis.
J. Phys. Soc. Jpn. 2006,
75, 034715.
[Google Scholar]
19.
Yanagisawa T, Miyazaki M, Yamaji K. Incommensurate antiferromagnetism coexisting with superconductivity in two-dimensional d–p model.
J. Phys. Soc. Jpn. 2009,
78, 031706.
[Google Scholar]
20.
Weber C, Lauchi A, Mila F, Giamarchi T. Orbital currents in extended Hubbard models of high-
Tc cuprate superconductors.
Phys. Rev. Lett. 2009,
102, 017005.
[Google Scholar]
21.
Lau B, Berciu M, Sawatzky GA. High-spin polaron in lightly doped CuO
2 planes.
Phys. Rev. Lett. 2011,
106, 036401.
[Google Scholar]
22.
Weber C, Giamarchi T, Varma CM. Phase diagram of a three-orbital model for high-
Tc cuprate superconductors.
Phys. Rev. Lett. 2014,
112, 117001.
[Google Scholar]
23.
Avella A, Mancini F, Paolo F, Plekhano E. Emery vs. Hubbard model for cuprate superconductors: A composite operator method study.
Euro. Phys. J. B 2013,
86, 265.
[Google Scholar]
24.
Ebrahimnejad H, Sawatzky GA, Berciu M. Differences between the insulating limit quasiparticles of one-band and three-band cuprate models.
J. Phys. Condens. Matter 2016,
28, 105603.
[Google Scholar]
25.
Tamura S, Yokoyama H. Variational study of magnetic ordered state in
d-
p model.
Phys. Procedia 2016,
81, 5–8.
[Google Scholar]
26.
Yanagisawa T, Miyazaki M, Yamaji K. Ground-state phase diagram of the three-band d-p model.
EPL 2021,
134, 27004.
[Google Scholar]
27.
Hubbard J. Electron correlations in narrow energy bands.
Proc. Roy. Soc. Lond. 1963,
276, 238–257.
[Google Scholar]
28.
Hubbard J. Electron correlations in narrow energy bands III. An improved solution.
Proc. Roy. Soc. Lond. 1964,
281, 401–419.
[Google Scholar]
29.
Gutzwiller MC. Effect of correlation on the ferromagnetism of transition metals.
Phys. Rev. Lett. 1963,
10, 159.
[Google Scholar]
30.
Zhang S, Carlson J, Gubernatis JE. Constrained path Monte Carlo method for fermion ground states.
Phys. Rev. B 1997,
55, 7464.
[Google Scholar]
31.
Zhang S, Carlson J, Gubernatis JE. Pairing correlations in the two-dimensional Hubbard model.
Phys. Rev. Lett. 1997,
78, 4486.
[Google Scholar]
32.
Yanagisawa T, Shimoi Y. Exact results in strongly correlated electrons—Spin-reflection positivity and the Perron-Frobenius theorem.
Int. J. Mod. Phys. B 1996,
10, 3383–3450.
[Google Scholar]
33.
Yanagisawa T, Shimoi Y, Yamaji K. Superconducting phase of a two-chain Hubbard model.
Phys. Rev. B 1995,
52, R3860.
[Google Scholar]
34.
Nakanishi T, Yamaji K, Yanagisawa T. Variational Monte Carlo indications of d-wave superconductivity in the two-dimensional Hubbard model.
J. Phys. Soc. Jpn. 1997,
66, 294–297.
[Google Scholar]
35.
Yamaji K, Yanagisawa T, Nakanishi T, Koike S. Variational Monte Carlo study on the superconductivity in the two-dimensional Hubbard model.
Physica C 1998,
304, 225–238.
[Google Scholar]
36.
Yamaji K, Yanagisawa T, Koike S. Bulk limit of superconducting condensation energy in 2D Hubbard model.
Physica B 2000,
284–288, 415–416.
[Google Scholar]
37.
Yamaji K, Yanagisawa T, Miyazaki M, Kadono R. Superconducting condensation energy of the two-dimensional Hubbard model in the large-negative-t’region.
J. Phys. Soc. Jpn. 2011,
80, 083702.
[Google Scholar]
38.
Hardy TM, Hague P, Samson JH, Alexandrov AS. Superconductivity in a Hubbard-Fröhlich model and in cuprates.
Phys. Rev. B 2009,
79, 212501.
[Google Scholar]
39.
Yanagisawa T, Miyazaki M, Yamaji K. Correlated Electrons and High-Temperature Superconductivity.
J. Mod. Phys. 2013,
4, 33–64.
[Google Scholar]
40.
Bulut N. dx2-y2 superconductivity and the hubbard model.
Adv. Phys. 2002,
51, 1587–1667.
[Google Scholar]
41.
Yokoyama H, Tanaka Y, Ogata M, Tsuchiura H. Crossover of superconducting properties and kinetic-energy gain in two-dimensional Hubbard model.
J. Phys. Soc. Jpn. 2004,
73, 1119–1122.
[Google Scholar]
42.
Yokoyama H, Ogata M, Tanaka Y. Mott transitions and d-wave superconductivity in half-filled-band Hubbard model on square lattice with geometric frustration.
J. Phys. Soc. Jpn. 2006,
75, 114706.
[Google Scholar]
43.
Miyazaki M, Yanagisawa T, Yamaji K. Possible coexistence of superconductivity and static SDW stripes in the two-dimensional Hubbard model.
J. Phys. Chem. Solids 2002,
63, 1403–1407.
[Google Scholar]
44.
Yanagisawa T. Phase diagram of the
t–
U2 Hamiltonian of the weak coupling Hubbard model.
New J. Phys. 2008,
10, 023014.
[Google Scholar]
45.
Yanagisawa T. Enhanced pair correlation functions in the two-dimensional Hubbard model.
New J. Phys. 2013,
15, 033012.
[Google Scholar]
46.
Yanagisawa T, Koike S, Yamaji K. Off-diagonal wave function Monte Carlo Studies of Hubbard model I.
J. Phys. Soc. Jpn. 1998,
67, 3867–3874.
[Google Scholar]
47.
Yanagisawa T. Crossover from Weakly to Strongly Correlated Regions in the Two-dimensional Hubbard Model—Off-diagonal Wave Function Monte Carlo Studies of Hubbard Model II—.
J. Phys. Soc. Jpn. 2016,
85, 114707.
[Google Scholar]
48.
Yanagisawa T. Antiferromagnetism, Superconductivity and Phase Diagram in the Two-Dimensional Hubbard Model—Off-Diagonal Wave Function Monte Carlo Studies of Hubbard Model III—.
J. Phys. Soc. Jpn. 2019,
88, 054702.
[Google Scholar]
49.
Yanagisawa T. Mechanism of high-temperature superconductivity in correlated-electron systems.
Condens. Matter 2019,
4, 57.
[Google Scholar]
50.
Noack RM, White SR, Scalapino DJ. The doped two-chain Hubbard model.
EPL 1995,
30, 163.
[Google Scholar]
51.
Noack RM, Bulut N, Scalapino DJ, Zacher MG. Enhanced d
x2−y2 pairing correlations in the two-leg Hubbard ladder.
Phys. Rev. B 1997,
56, 7162.
[Google Scholar]
52.
Yamaji K, Shimoi Y, Yanagisawa T. Superconductivity indications of the two-chain Hubbard model due to the two-band effect.
Physica C 1994,
235, 2221–2222.
[Google Scholar]
53.
Koike S, Yamaji K, Yanagisawa T. Effect of the medium-range transfer energies to the superconductivity in the two-chain Hubbard model.
J. Phys. Soc. Jpn. 1999,
68, 1657–1663.
[Google Scholar]
54.
Yamaji K, Harigaya K, Yanagisawa T, Shimoi Y. Superconducting phase diagram of the two-band model investigated by the exact diagonalization method.
J. Phys. Soc. Jpn. 1992,
61, 3689–3698.
[Google Scholar]
55.
Nakano T, Kuroki K, Onari S. Superconductivity due to spin fluctuations originating from multiple Fermi surfaces in the double chain superconductor Pr
2Ba
4Cu
7O
15−δ.
Phys. Rev. B 2007,
76, 014515.
[Google Scholar]
56.
Tranquada JM, Axe JD, Ichikawa N, Nakamura Y, Uchida S, Nachumi B. Neutron-scattering study of stripe-phase order of holes and spins in La
1.48Nd
0.4Sr
0.12CuO
4.
Phys. Rev. B 1996,
54, 7489.
[Google Scholar]
57.
Suzuki T, Goto T, Chiba K, Shinoda T, Fukase T, Kimura H, et al. Observation of modulated magnetic long-range order in La
1.88Sr
0.12CuO
4.
Phys. Rev. B 1998,
57, R3229.
[Google Scholar]
58.
Yamada K, Lee CH, Kurahashi K, Wada J, Wakimoto S, Ueki S, et al. Doping dependence of the spatially modulated dynamical spin correlations and the superconducting-transition temperature in La
2−xSr
xCuO
4.
Phys. Rev. B 1998,
57, 6165.
[Google Scholar]
59.
Arai M, Nishijima T, Endoh Y, Egami T, Tajima S, Tomimoto K, et al. Incommensurate spin dynamics of underdoped superconductor YBa
2Cu
3O
6.7.
Phys. Rev. Lett. 1999,
83, 608.
[Google Scholar]
60.
Mook HA, Dai P, Doga F, Hunt RD. One-dimensional nature of the magnetic fluctuations in YBa
2Cu
3O
6.6.
Nature 2000,
404, 729–731.
[Google Scholar]
61.
Wakimoto S, Birgeneau RJ, Kastner MA, Lee YS, Erwin R, Gehring PM, et al. Direct observation of a one-dimensional static spin modulation in insulating La
1.95Sr
0.05CuO
4.
Phys. Rev. B 2000,
61, 3699.
[Google Scholar]
62.
Bianconi A, Saini NL, Lanzara A, Missori M, Rossetti T, Oyanagi H, et al. Determination of the Local Lattice Distortions in the CuO2 Plane of La
1.85Sr
0.15CuO
4.
Phys. Rev. Lett. 1996,
76, 3412.
[Google Scholar]
63.
Kato M, Machida K, Nakanishi H, Fujita M. Soliton lattice modulation of incommensurate spin density wave in two dimensional Hubbard model-a mean field study.
J. Phys. Soc. Jpn. 1990,
59, 1047–1058.
[Google Scholar]
64.
Maier TA, Alvarez G, Summers M, Schulthess TC. Dynamic Cluster Quantum Monte Carlo Simulations of a Two-Dimensional Hubbard Model with Stripelike Charge-Density-Wave Modulations: Interplay between Inhomogeneities and the Superconducting State.
Phys. Rev. Lett. 2010,
104, 247001.
[Google Scholar]
65.
Mondaini R, Ying T, Paiva T, Scalettar RT. Determinant quantum Monte Carlo study of the enhancement of d-wave pairing by charge inhomogeneity.
Phys. Rev. B 2012,
86, 184506.
[Google Scholar]
66.
Bianconi A. Shape resonances in superstripes.
Nat. Phys. 2013,
9, 536–537.
[Google Scholar]
67.
Yamase H, Sakurai Y, Fujita M, Wakimoto S, Yamada K. Fermi surface in La-based cuprate superconductors from Compton scattering imaging.
Nature Commun. 2021,
12, 2223.
[Google Scholar]
68.
Miyazaki M, Yanagisawa T. Ferromagnetic diagonal stripe states in the two-dimensional Hubbard model with U ≲ ∞.
Phys. Lett. A 2022,
446, 128276.
[Google Scholar]
69.
Yanagisawa T, Koike S, Miyazaki M, Yamaji K. Stripe formation in high-
Tc superconductors.
J. Phys. Condens. Matter 2001,
14, 21.
[Google Scholar]
70.
Ying T, Mondaini R, Sun XD, Paiva T, Fye RM, Scalettar RT. Determinant quantum Monte Carlo study of d-wave pairing in the plaquette Hubbard hamiltonian.
Phys. Rev. B 2014,
90, 075121.
[Google Scholar]
71.
Yang S, Ying T, Li W, Yang J, Sun X, Li X. Quantum Monte Carlo study of the Hubbard model with next-nearest-neighbor hopping
t′: Pairing and magnetism.
J. Phys. Condens. Matter 2021,
33, 115601.
[Google Scholar]
72.
Hoffman JE, McElroy K, Lee D-H, Lang KM, Eisaki H, Uchida S, et al. A four unit cell periodic pattern of quasi-particle states surrounding vortex cores in Bi
2Sr
2CaCu
2O
8+δ.
Science 2002,
295, 466–469.
[Google Scholar]
73.
Wise WD, Boyer MC, Chatterjee K, Kondo T, Takeuchi T, Ikuta H, et al. Charge-density-wave origin of cuprate checkerboard visualized by scanning tunnelling microscopy.
Nat. Phys. 2008,
4, 696–699.
[Google Scholar]
74.
Hanaguri T, Lupien C, Kohsaka Y, Lee D-H, Azuma M, Takano M, et al. A checkerboard electronic crystal state in lightly hole-doped Ca
2-xNa
xCuO
2Cl
2.
Nature 2004,
430, 1001–1005.
[Google Scholar]
75.
Miyazaki M, Yanagisawa T, Yamaji K. Checkerboard states in the two-dimensional Hubbard model with the Bi2212-type band. Journal of the Physical Society of Japan.
J. Phys Soc. Jpn. 2009,
78, 043706.
[Google Scholar]
76.
Valletta A, Bianconi A, Perali A, Saini NL. Electronic and superconducting properties of a superlattice of quantum stripes at the atomic limit.
Z. Phys. B Condens. Matter 1997,
104, 707–713.
[Google Scholar]
77.
Shirage PM, Kihou K, Miyazawa K, Lee C-H, Kito H, Eisaki H, et al. Inverse iron isotope effect on the transition temperature of the (Ba, K) Fe
2As
2 superconductor.
Phys. Rev. Lett. 2009,
103, 257003.
[Google Scholar]
78.
Yanagisawa T, Odagiri K, Hase I, Yamaji K, Shirage P, Tanaka Y, et al. Isotope effect in multi-band and multi-channel attractive systems and inverse isotope effect in iron-based superconductors.
J. Phys. Soc. Jpn. 2009,
78, 094718.
[Google Scholar]
79.
Perali A, Innocenti D, Valletta A, Bianconi A. Anomalous isotope effect near a 2.5 Lifshitz transition in a multi-band multi-condensate superconductor made of a superlattice of stripes. Superconductor Science and Technology.
Superconduct. Sci. Technol. 2012,
25, 124002.
[Google Scholar]
80.
Perali A, Bianconi A, Lanzara A, Saini NL. Lanzara and NL Saini.
Solid State Commun. 1996,
100, 181.
[Google Scholar]
81.
Bianconi A, Valletta A, Perali A, Saini NL. Superconductivity of a striped phase at the atomic limit.
Physica C 1998,
296, 269–280.
[Google Scholar]
82.
Kusmatsev FV, Di Castro D, Bianconi G, Bianconi A. Transformation of strings into an inhomogeneous phase of stripes and itinerant carriers.
Phys. Lett. A 2000,
275, 118–123.
[Google Scholar]
83.
Müller KA, Zao GM, Conder K, Keller H. The ratio of small polarons to free carriers in derived from susceptibility measurements.
J. Phys. Condens. Matter 1998,
10, L291.
[Google Scholar]
84.
Bianconi A. On the Fermi liquid coupled with a generalized Wigner polaronic CDW giving high Tc superconductivity.
Solid State Commun. 1994,
91, 1.
[Google Scholar]
85.
Feiner LF, Jefferson JH, Raimondi R. Effective single-band models for the high-
Tc cuprates. I. Coulomb interactions.
Phys. Rev. B 1996,
53, 8751.
[Google Scholar]
86.
Kuroki K, Onari S, Arita R, Usui H, Tanaka Y, Kontani H, et al. Unconventional Pairing Originating from the Disconnected Fermi Surfaces of Superconducting LaFeAsO
1−xF
x.
Phys. Rev. Lett. 2008,
101, 087004.
[Google Scholar]
87.
Grewe N, Steglich F. Handbook on the Physics and Chemistry of Rare Earths; Elsevier Science B. V.: Amsterdam, The Netherland, 1991; Volume 14, p. 343.
88.
Hewson AC. The Kondo Problem to Heavy Fermions; Cambridge University Press: Cambridge, UK, 1993.
89.
Onuki Y. Physics of Heavy Fermions: Heavy Fermions and Strongly Correlated Electron Systems; World Scientific Pub Co Inc.: Singapore, 2018.
90.
Ishiguro T, Yamaji K, Saito G. Organic Superconductors; Springer: Berlin/Heidelberg, Germany, 2012.
91.
Akashi R, Kawamura M, Tsuneyuki S, Nomura Y, Arita R. First-principles study of the pressure and crystal-structure dependences of the superconducting transition temperature in compressed sulfur hydrides.
Phys. Rev. B 2015,
91, 224513.
[Google Scholar]
92.
Otsuka H. Variational Monte Carlo Studies of the Hubbard Model in One-and Two-Dimensions—Off-Diagonal Intersite Correlation Effects—.
J. Phys. Soc. Jpn. 1992,
61, 1645–1656.
[Google Scholar]
93.
Yanagisawa T, Koike S, Yamaji K.
d-wave state with multiplicative correlation factors for the Hubbard model.
J. Phys. Soc. Jpn. 1999,
68, 3608–3614.
[Google Scholar]
94.
Eichenberger D, Bäriswyl D. Superconductivity and antiferromagnetism in the two-dimensional Hubbard model: A variational study.
Phys. Rev. B 2007,
76, 180504.
[Google Scholar]
95.
Bäriswyl D, Eichenberger D, Menteshashvii M. Variational ground states of the two-dimensional Hubbard model.
New J. Phys. 2009,
11, 075010.
[Google Scholar]
96.
Bäriswyl D. Superconductivity in the repulsive Hubbard model.
J. Supercond. Novel Magn. 2011,
24, 1157.
[Google Scholar]
97.
Yanagisawa T, Miyazaki M. Mott transition in cuprate high-temperature superconductors.
EPL 2014,
107, 27004.
[Google Scholar]
98.
Anderson PW. The resonating valence bond state in La
2CuO
4 and superconductivity.
Science 1987,
235, 1196–1198.
[Google Scholar]
99.
Yanagisawa T. Quantum Monte Carlo diagonalization for many-fermion systems.
Phys. Rev. B 2007,
75, 224503.
[Google Scholar]
100.
Wilson KG. The renormalization group: Critical phenomena and the Kondo problem.
Rev. Mod. Phys. 1975,
47, 773.
[Google Scholar]
101.
Loram JW, Mirza KA, Cooper JR, Liang WY. Electronic specific heat of YBa
2Cu
3O
6+x from 1.8 to 300 K.
Phys. Rev. Lett. 1993,
71, 1740.
[Google Scholar]
102.
Hao Z, Clem JR, McElfresh MW, Civale L, Malozemoff AP, Holtzberg F. Model for the reversible magnetization of high-κ type-II superconductors: Application to high-
Tc superconductors.
Phys. Rev. B 1991,
43, 2844.
[Google Scholar]
103.
Maier TA, Jarrell M, Macridin A, Slezak C. Kinetic energy driven pairing in cuprate superconductors.
Phys. Rev. Lett. 2004,
92, 027005.
[Google Scholar]
104.
Ogata M, Yokoyama H, Yanase Y, Tanaka Y, Tsuchiura H. Kinetic-energy pairing and condensation energy in cuprates.
J. Phys. Chem. Solids 2006,
67, 37–40.
[Google Scholar]
105.
Gull E, Millis AJ. Energetics of superconductivity in the two-dimensional Hubbard model.
Phys. Rev. B 2012,
86, 241106.
[Google Scholar]
106.
Tocchio LF, Becca F, Sorella S. Hidden Mott transition and large-U superconductivity in the two-dimensional Hubbard model.
Phys. Rev. B 2016,
94, 195126.
[Google Scholar]
107.
Feng S. Kinetic energy driven superconductivity in doped cuprates.
Phys. Rev. B 2003,
68, 184501.
[Google Scholar]
108.
Wrobel P, Eder R, Micnas R. Kinetic energy driven superconductivity and the pseudogap phase in weakly doped antiferromagnets.
J. Phys. Condens. Matter 2003,
15, 2755.
[Google Scholar]
109.
Guo H, Feng S. Electronic structure of kinetic energy driven superconductors.
Phys. Lett. A 2007,
361, 382–390.
[Google Scholar]
110.
Yanagisawa T, Miyazaki M, Yamaji K. On the Kinetic Energy Driven Superconductivity in the Two-Dimensional Hubbard Model.
Condens. Matter 2021,
6, 12.
[Google Scholar]
111.
Yanagisawa T. Enhancement of superconductivity due to kinetic-energy effect in the strongly correlated phase of the two-dimensional Hubbard model.
Phys. Lett. A 2021,
403, 127382.
[Google Scholar]
112.
Deutscher G, Santander-Syro AF, Bontemps N. Kinetic energy change with doping upon superfluid condensation in high-temperature superconductors.
Phys. Rev. B 2005,
72, 092504.
[Google Scholar]
