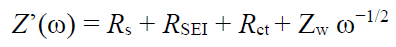Lithium-Rich Spinel Cathode with Higher Energy Density for Sustainable Li-Ion Batteries Operating in Extended Potential Range

3.1. Structural and Compositional Properties XRD pattern for the LMO900 sample synthesized by sol-gel method using EDTA as a chelating agent at annealing temperature of 900 °C is shown in Figure 2a. In the 2θ range 10–80°, the XRD patterns reveal eight intense peaks located at 18.7°, 36.1°, 37.7°, 43.7°, 48.1°, 58.1°, 63.8°, and 67.1°, which correspond to the (111), (311), (222), (400), (422), (511), (440), and (531) crystal planes of the LiMn₂O₄ spinel phase, respectively. The XRD reflections of the LMO material calcined at 900 ºC are indexed according to the single spinel LiMn₂O₄ phase with cubic structure $$Fd\bar{3}m$$ (O_h⁷) (JCPDS card No. 35-0782). The diffraction peaks corresponding to the (111), (311), and (400) lattice planes serve as the primary features of the spinel LiMn₂O₄ phase. In this phase, Li ions occupy the tetrahedral 8a sites (Wyckoff notation), Mn ions are distributed at octahedral 16d sites, and oxygen anions are located at the 32e sites [7]. Notably, the pure spinel phase is achieved during the transformation of the solid Mn₃O₄ → LiMn₂O₄ after calcination at 900 °C. The cubic lattice parameter a was calculated via the least square method using 10 well-defined reflections with indexation in the cubic structure. The crystallographic results summarized in Table 1 match well with the literature data [38,39,40,41,42,43]. The intensity ratio of R_int = I(₃₁₁)/I(₄₀₀) is thought to be closely related to the electrochemical performance of spinel LiMn₂O₄. LMO900, with R_int = 0.87, is expected to exhibit good electrochemical performances. In contrast, samples heat treated at 450 and 750 °C display additional reflections at 2θ ≈ 32° attributed to Mn₃O₄ impurities in their XRD patterns. The peaks associated with the LiMn₂O₄ spinel phase are well-defined but slightly broadened, indicating the formation of nanosized grains with significant lattice strain. These features do not satisfy the structural criterion of an optimized electrode and are unfavorable for high electrochemical performance to attain high-rate capability and cycling stability [38]. Raising the annealing temperature to 900 °C results in a gradual sharpening of all peaks, signifying an enhancement in crystallinity with local structure ordering, grain size growth, and/or release of lattice strain. The lattice parameter slightly increases from 8.2322 to 8.2449 Å during calcination from 700 to 900 °C. Additionally, the structure and composition of the LMO900 sample have been examined through Rietveld refinement (Figure 2a). The small values of the residual and reliability factors (R_p = 8.36%, R_wp = 11.87%, and χ² = 1.41) in the Rietveld analysis indicate the successful identification of the cubic phase. The difference between the calculated and experimental diffractogram (blue curve) demonstrates the quality of the fit, validating the structural model. Results indicate an under-occupancy of Mn and a smaller unit-cell parameter (a = 8.2449 Å) compared to that for a perfect spinel structure for stoichiometric LiMn₂O₄ (a₀ = 8.24762 Å and V₀ = 561.03 ų). This suggests that the LMO900 sample is Li doped and has the composition Li_1.03Mn_1.97O_3.99 with 3 mol.% excess of Li. This composition implies the substitution of excess Li ions onto the Mn 16d site in the spinel, resulting in an average Mn oxidation state of 3.54 [17]. Additional structural parameters are worth considering to quantify the residual lattice strain and describe the slight variation (if it exists) caused by increased calcination temperature. First, the full-width at half-maximum (FWHM) of the (400) peak at 2θ = 43.7° is highly sensitive to both the calcination temperature and the degree of crystallization and local structural order. Therefore, as reported by several reports, the lower value of the FWHM of the (400) peak is related to higher crystallinity and better ordering of the local structure [30,31]. It is noted that the FWHM of the (400) peak decreases with increasing calcination temperature, indicating that both crystallinity and local structure ordering of the LMO900 sample is the largest among all samples. cubic lattice parameters and crystallite size increase. The lattice parameter increase is attributed to a significant expansion of the spinel lattice, which matches well with previous LiMn₂O₄ reports [29,32], while the increase in the crystallite size may be due to a residual lattice strain. Broadening of diffraction peaks is the third fingerprint of the crystallinity of LiMn₂O₄ powders and the homogeneous distribution of cations within the structure. The microstrain (ε) of the LMO900 particles was determined using the Williamson-Hall equation [44]: where B_hkl is the line broadening of a Bragg reflection (hkl), K = 0.9 is the shape factor for a spherical particle, L_c is the crystallite size, and λ is the X-ray wavelength. The microstrain is estimated from the slope of the plot (B_hkl cos θ_hkl) vs. (4sinθ_hkl), and the intersection with the vertical axis provides the crystallite size (Figure 2b). The B_hkl value used here is the instrumental corrected one. The microstrain (ε) is inversely proportional to the calcination temperature. The small value of the LMO900 microstrain ε = 0.72 × 10⁻³ rd depicts that the local field strain was decreased as a result of lattice ordering upon calcination at 900 °C. It can be compared to ε = 1.5 × 10⁻³ rd for LiMn₂O₄ synthesized via two-step precipitation method [45], to ε = 10.7 × 10⁻⁴ rd for LiMn₂O₄ prepared via rapid calcination of oxalates at 800 °C [46], and to ε = 1.3 × 10⁻³ rd for LiMn₂O₄ prepared through solid-state reaction of the MnO+Li₂CO₃ mixture [47]. This result is consistent with the crystallite size that was previously discussed. Comparing the lattice parameter a, FWHM of (400) peak, strain values, and I(₃₁₁)/I(₄₀₀) ratio value of spinel lithium manganese oxides obtained by sol-gel method at different temperatures, we can conclude that the sample (LMO900) prepared at high temperature is well crystallized. This sample exhibits a crystallite size of 65 ± 1 nm determined by the Scherrer formula and shows a lower degree of particle agglomeration due to the synergetic effect of the post-annealing. LMO synthesized at 900 °C is expected to show better electrochemical performance than samples calcined at lower temperatures [48]. 3.2. Thermal Analysis In order to obtain complementary information on the exact temperature of the phase formation reaction of the spinel phase, we have performed a combined thermal gravimetry analysis (TGA) and differential thermal analysis (DTA) on the dried precursor complex in the temperature range 30–1000 °C. The results are shown in Figure 3a. The TGA curve shows two distinguishable transformation enthalpies, i.e., two weight loss steps occurring at ca. 100 and 235 °C. The endotherm observed at about 100 °C accompanied by a noticeable weight loss (3.1%), is attributed to the evaporation of residual water due to the hygroscopic nature of the precursor sample. As the heating process continues, an exothermic transformation begins to appear at around 235 °C, indicating the onset of the decomposition and/or the soft oxidation of the molecular precursor [49]. At 378 °C, a significant exothermic reaction occurs due to the decomposition of the organic moieties present in the precursor complex (attributed to the EDTA agent). This reaction occurs in the presence of atmospheric oxygen (in the air) and leads to a weight loss of over 50%s due to a violent oxidation-decomposition reaction. Eventually, this reaction results in the formation of the spinel structure. In this reaction, EDTA seems to function as a fuel in the pyrolysis of the molecular precursors, accelerating the decomposition process of all reactants. This behavior is characteristic of wet-chemistry synthesis using chelating agents such as glycolic, adipic, and oxalic acid [50,51]. The spinel phase begins to form at 370 °C due to the synergistic effect of all the reactants (lithium acetate and manganese acetate), as evidenced by the XRD analysis. The spinel remains stable from nearly 370 °C to 930 °C without any observable further weight loss. The stability of the spinel structure is confirmed by the TG analysis of the as-prepared LMO900 sample (Figure 3b). No distinct weight loss in the TG curve is observed until 900 °C, indicating that the spinel phase formed by the sol-gel method using EDTA as a chelating agent has a stable structure. This stability has been reported in the literature [52]. Only slight weight loss of ~4% occurs above 900 °C, which may be attributed to the volatilization and liberation of lithium at such high temperatures. The concentration of the different elements deduced from the EDX spectrum shown in Figure 3c (O/M = 2.025) is consistent with results from Rietveld refinements. 3.3. Morphological Properties It is well known that particle size, surface morphology, and particle distribution are crucial factors influencing Li-ion battery performance. The electron microscopy analyses (SEM, TEM and HRTEM) of the LMO900 sample are presented in Figure 4 and Figure 5, respectively. The SEM image (Figure 4a) reveals well-crystallized particles with similar morphology (almost monodisperse). LMO900 particles present a smooth surface with noticeable edges and corners. This material exhibits a truncated octahedral structure with a face-center cubic (fcc) framework. The particle size distribution was evaluated using the ImageJ software. SEM image analysis was conducted on approximately a hundred nanoparticles. The particles are uniformly dispersed with average size of ~380 nm, as shown in Figure 4b. These results indicate that the calcination process at 900 °C is beneficial for the growth of single-phase LMO crystals. Additionally, calcining at 900 °C combined with using EDTA as a chelating agent encourages the selective growth of the (110) and (100) crystal planes in LMO materials. This expands the diffusion channels for lithium ions, enhancing the material’s electrochemical performance.The particles appear relatively large compared to spinel LiMn₂O₄ particles prepared by other synthesis methods [45,49], likely due to aggregation and agglomeration effects caused by the use of EDTA as a fuel, as illustrated before. The use of EDTA increases the surface energy during the calcination process, causing the crystalline grains to be adjacent and susceptible to anomalous growth. Features of truncated octahedral LiMn₂O₄ with face-center cubic structure, mainly exposing (111) planes and truncated with (100) and (110) planes, have been previously reported by several researchers [53,54,55,56]. They argue that the (111) planes, with thedensest Mn arrangements, are prone to form stable SEI layers, thereby suppressing Mn dissolution in the electrolyte. The tap density was determined by manually filling a graduated cylinder with the sample, followed by tapping to the constant volume of the sample in the cylinder. The tapped density of 1.85 g cm⁻³ was calculated from the ratio between the sample mass and its final volume. To gain a deeper understanding of the structural information, the morphology and surface state of LMO particles were examined in detail by transmission electron microscopy (TEM). Figure 5a shows the TEM image of the LMO900 sample, further confirming the truncated octahedral structure with a uniform size distribution. The corresponding HRTEM images (Figure 5b,c) clearly exhibit lattice fringes with the d spacing of 0.457 nm, which matches well with the inter-planar distance of the (111) of LMO, indicating remarkable structural compatibility [46,47]. Therefore, LMO900 exhibits well-defined fringes, indicating the good crystallinity of powder. It has been reported that dominant (111) surfaces minimize Mn dissolution, facilitate Li⁺ ions insertion/desertion, and reduce structural and volume change. Therefore, it can provide a superior cycle life and facilitate high discharge rate capabilities [57]. The related FFT pattern agrees well with the selected SAED pattern of the whole particle of LMO900 and shows well-defined spots, revealing its single crystalline nature (Figure 5d). The porosity of the synthesized LMO900 sample was investigated using the BET technique. Figure 6 presents the N₂ adsorption/desorption isotherm and the pore-size distribution (inset) for the LMO900 sample as a function of the relative pressure (P/P₀, P₀ is standard atmosphere pressure) measured at 77 K. The isotherm curves exhibit an increase in the amount of adsorbed N₂ with the rise in the P/P₀ value, accompanied by a hysteresis loop. This isotherm profile can be categorized as a type-IV curve with an H3-hysteresis loop, according to the IUPAC classification, implying the existence of a large number of mesopores. BET analysis shows that the LMO900 sample has a specific surface area of 1.68 m² g⁻¹. This value is larger than previously obtained for LiMn₂O₄ synthesized at the same temperature via evaporation method-assisted oxalic acid as a precipitating agent [49]. Using the Barrett-Joyner-Halenda (BJH) model (via DFT method), the pore volume and size distribution were derived from the adsorption branches of the isotherms, and the total pore volumes were estimated from the adsorbed amount at a relative pressure P/P₀ of 0.995. As shown in the insets of Figure 6, the BJH pore size distribution reveals that the LMO900 sample possesses a mesoporous character, with monodisperse mesopore sizes estimated to be 5.1 nm with a total pore volume of 2.8 × 10⁻³ cm³ g⁻¹. These features may be beneficial, allowing the electrolyte to fully penetrate the pores and facilitating efficient diffusion to active sites with minimal resistance. Additionally, they can buffer the large volume changes during Li-ion insertion/extraction processes. Moreover, the uniformly interconnected mesopore size can decrease diffusion lengths by approximately 10 nm [58]. Note that particle size (L_BET = 415 nm) can also be calculated from BET measurements by using the relationship A = 3/(ρL_BET), where A is the specific surface area, ρ is the density (4.3 g cm⁻³ for LiMn₂O₄). 3.4. Electronic State Analysis The electronic states of the elements in the spinel sample were revealed by X-ray photoelectron spectroscopy, as presented in Figure 7. The survey spectrum (Figure 7a) of the LMO900 specimen shows peaks with Gaussian-like line shape at binding energies of 84.5 eV for Mn 3s, 530.5 eV for O 1s, 55.0 eV for Li 1s, 642.3 eV for Mn 2p_3/2, and 654.4 eV for Mn 2p_1/2, which match well with the characteristic XPS patterns of the LiMn₂O₄ spinel phase [59]. The high-resolution XPS spectra of the Mn 2p, Mn 3s, and O 1s core levels for the spinel LMO900 sample are illustrated in Figure 7b–d, respectively. The experimental binding energies were corrected for specimen charging by referencing the C 1s line at 284.5 eV. As shown in Figure 7b, the Mn 2p_3/2 and Mn 2p_1/2 doublet of Mn 2p (with 11.6 eV spin-orbital energy separation) can be fitted in two bands corresponding to the binding position of Mn³⁺ and Mn⁴⁺ cations. The XPS spectrum of O 1s shown in Figure 7 corresponds to the (O-M-O) bonds. A shoulder peak observed at 530.9 eV is assigned to the residual oxygen related to impurities with OH⁻ or O⁻ bonding on the surface [60]. In order to accurately calculate the Mn³⁺ and Mn⁴⁺ contents, specific peaks of Mn 2p_3/2 were fitted and the results are shown in Figure 7b and listed in Table 2. The average Mn valence state is 3.53 due to 53.2% Mn⁴⁺ and 46.8% Mn³⁺. Also, the exchange splitting value (ΔE_3s) of Mn 3s can be used to determine the average valence state of Mn via a more quantitative method. According to Beyreuther et al. [61], there is a linear relationship between the Mn exchange splitting energy and Mn valence governed by the equation (ν_Mn = 9.67−1.27 ΔE_3s/eV), which is used to approximately estimate the average valence state of Mn. Figure 7c shows that the ΔE_3s for LMO900 sample is ≈4.83 eV, corresponding to an average Mn valence state of 3.54. This result means that the excess 3 mol.% of Li is able to reduce the active J–T Mn³⁺ ion, i.e., 3 mol.% of Mn³⁺ is replaced by Li⁺ on the Mn 16d site in the spinel framework. XPS results are consistent with the XRD data of the lithium-rich Li_1.03Mn_1.97O_3.99 phase. 3.5. Electrochemical Performance Unlike previously published reports on Li-Mn-O spinels in the 4-V region [45,49,62], this work investigates the electrochemical properties of spinel Li_1.03Mn_1.97O_3.99 in both a narrow (3.0–4.5 V) and an extended (1.5–4.8 V) potential window using cyclic voltammetry (CV) and galvanostatic charge-discharge (GCD) method. Figure 8a displays the CV curves of the LMO900 electrode in the conventional voltage range of 3.0–4.5 V vs. Li⁺/Li at a sweep rate of 0.1 mV s⁻¹ over 4 cycles. All voltammograms exhibit two well-defined, separated reversible redox peaks at ~ 4.07/3.87 V and ~ 4.21/3.99 V. These peaks are associated with the reversible ingress and egress of Li⁺ ions into/from the tetrahedral sites (8a) of the spinel lattice. Bianchini et al. [40] explored the phase diagram of a series of Li-Mn-O spinels. Stoichiometric LiMn₂O₄ undergoes two biphasic reactions, represented by the α + β and β + ε transitions, with ε < 0.1 close to λ-Mn₂O₄, with the intermediate β-phase close to Li_0.5Mn₂O₄ [63]. The same behavior is observed for the Li-rich compound Li_1.03Mn_1.97O_3.99, where the total amount of extractable lithium decreases as 1 − 3x = 0.91. Therefore the amount of lithium that remains in the structure at the end of the charge increases to 0.12 (i.e., reaching the fully oxidized Mn^IV composition Li_0.12Mn_1.97O_3.99). On the other hand, Figure 8b displays the CV curves of the LMO900 material within the wide potential range of 1.5−4.8 V vs. Li⁺/Li, using the same scan rate of 0.1 mV s⁻¹ over 6 cycles. The peaks become well-defined and sharper than those cycled at the narrow voltage window (3.0−4.5 V), and the high current response at the wider voltage window was observed. Additionally, it has higher oxidation and reduction current peaks and a larger area, demonstrating the optimal reversibility of Li-ion transportation (i.e., Coulombic efficiency) and superior specific capacity. Besides the two redox peaks characteristic of the cubic stoichiometric spinel LiMn₂O₄ ($$Fd\bar{3}m$$ s.g.), there is notably the presence of an additional redox peak below the 3-V region at ~3.3/2.6 V, which remains stable over cycling. This additional redox reaction is a feature of the Li-ion storage at octahedral sites (16c), involving the conversion of cubic (LiMn₂O₄ (to tetragonal phase transition (Li₂Mn₂O₄) [23,64]. This transformation results from an overlithiation case, in which 50% of Mn⁴⁺:d³ (t_2g³) in the cubic structure converts to high spin Mn³⁺:d⁴(t_2g³eg¹). This transformation is also confirmed by the oxidation and reduction current peak ratios (Ipa/Ipc) [63]. An additional oxidation peak at 3.8 V is attributed in the literature to parasitic reactions [65,66]. The peak potential difference (ΔE_p) between the oxidation and reduction peaks can provide insights into the impedance growth related to electron transfer and the polarization degree of the battery [67]. The ΔE_p values of LMO900 cycled in the wide potential window 1.5–4.8 V are in the range 0.27–0.29 V, slightly larger than those of LMO900 cycled at the conventional potential range (0.19 V). This suggests that this sample may have a larger electrochemical reaction polarization, possibly due to the tetragonal phase (Li₂Mn₂O₄) presence. However, the sample exhibits similar lower potential intervals (ΔE_p) over the subsequent five cycles, suggesting good stable cycle performance. On the other hand, ΔE_p values in the conventional narrow voltage range of 3.0–4.5 V decrease with cycling, indicating the stability of the cubic phase and the improvement of reversibility due to fast Li⁺ ion transport. Therefore, we can conclude from the cyclic voltammograms of LMO900 that extra Li-ion storage at the 16c octahedral vacancies in spinel LiMn₂O₄ was more easily activated in the wide potential window (1.5–4.8 V), leading to the production of the tetragonal phase Li₂Mn₂O₄. This effect on the cycle performance will be investigated in detail in the following. The GCD profiles of the LMO900 electrode cycled at a current density of 100 mA g⁻¹ for 100 and 500 cycles in the potential range 3.0–4.5 V and 1.5–4.8 V vs. Li⁺/Li are shown in Figure 9a,b, respectively. During the first charging and discharging process, Figure 9a displays two distinct voltage charge plateaus at ~4.10/4.20 V vs. Li⁺/Li and two distinct voltage discharge plateaus at 4.05/3.94 V vs. Li⁺/Li, respectively. These plateaus corresponding to the LiMn₂O₄/Li_0.5Mn₂O₄ and Li_0.5Mn₂O₄/Li_εMn₂O₄ two-phase systems match well with the CV results. For LMO900 operating in the wide voltage range of 1.5–4.8 V (Figure 9b), a slight polarization can be detected after 100 cycles. Notably, the polarization decreases over cycling. In the first 5 subsequent cycles, both charge and discharge voltage plateaus align with each other and with CV peaks. An additional plateau appears at ~2.8 V in the wide voltage range 1.5–4.8 V, corresponding to the tetragonal phase Li₂Mn₂O₄ and generally assigned as “3-V plateau” in literature [64]. The additional plateaus are consistent during the first five cycles and correspond to CV peaks. When cycled 100 times in the wide potential range of 1.5–4.8 V, the additional plateau gradually disappears, indicating gradual conversion of the tetragonal phase Li₂Mn₂O₄ to the cubic LiMn₂O₄. This result is also evident in the charge profile, as the plateau starts to disappear and takes on the shape of a charge profile of the 3.0–4.5 V range, accompanied by a decrease in polarization with repeated cycling. Figure 9c,d illustrate the cycle stability and Coulombic efficiency of the LMO900 cathode material cycled at a current density of 100 mA g⁻¹ (approximately 0.7 C rate) within the narrow potential window 3.0–4.5 V and the extended potential window 1.5–4.8V vs. Li⁺/Li, respectively. Within the conventional voltage range of 3.0–4.5 V, the Li//LMO900 cell initially provides a specific capacity of 85 mAh g⁻¹, and after 100 cycles, it maintains 46 mAh g⁻¹. This corresponds to a capacity retention of 54.3%, with a significant capacity decay of 45.7% (equivalent to a capacity loss of approximately 0.46% per cycle). Remarkably, within the extended potential range of 1.5–4.8 V, the same cell exhibits an initial specific capacity of 173 mAh g⁻¹. After 100 cycles, it retains 123 mAh g⁻¹, resulting in a capacity retention of 71.45% and a minor capacity decay of 28.55%. The capacity loss is thus reduced to 0.28% per cycle. Note that such a cell provides a specific capacity of ~125 mAh g⁻¹ in the 3-V region. After 500 cycles, the Li//LMO900 cell gives 77 mAh g⁻¹ with a capacity retention of 44.6%. This test shows a small capacity decay of 0.15 mAh g⁻¹ per cycle. The findings suggest that a slight excess of Li in Mn 16d sites enhances specific capacity and reduces capacity loss by preventing J-T distortion in the Li-rich lattice.The observed increase in discharge capacity at lower voltage levels may be attributed to the presence of a tetragonal phase (Li₂Mn₂O₄), as the standard spinel (LiMn₂O₄) has a theoretical capacity of 148 mAh g⁻¹ for the insertion and extraction of 1 Li⁺ per formula unit. In Li₂Mn₂O₄, an overlithiation state (2 Li⁺ per formula unit) occurs, as Li ions can also insert into octahedral 16c sites, which are face-shared with tetrahedral 8a sites. This expanded capacity contributes to the increased discharge capacity observed in the lower voltage range. The representative GCD profiles of the LMO900 electrode tested in both potential domains at different current densities from 100 to 1000 mA g⁻¹ (with 1C = 148 mA g⁻¹) are depicted in Figure 10a,b. Even at high rates, the 4-volt plateaus remain recognizable. The GCD profiles of the spinel electrode cycled in the wide potential range show a remarkable phenomenon: the reduction of the plateau related to the tetragonal phase at 2.8 V (marked with an arrow in Figure 10b) until it disappears with increasing current density. This finding is attributed to the tetragonal phase behaving like a cubic phase under high current density conditions. Figure 10c shows the rate capability of the LMO900 electrode tested in the narrow potential range of 3.0–4.5 V. The discharge capacity values decrease with increasing C-rates in the sequence 85, 52, 35, 30, and 15 mAh g⁻¹ at 100, 200, 400, 500, and 1000 mA g⁻¹, respectively. When cycled again at 100 mA g⁻¹, the discharge capacity of the spinel sample reached 64.6 mAh g⁻¹ with a capacity retention of 76%. On the other side, LMO900 shows discharge capacity of 162, 135, 105, 83, and 58 mAh g⁻¹ (Figure 10d) when tested in the wide potential range 1.5–4.8 V using the same current densities at 100, 200, 400, 500 and 1000 mA g⁻¹, respectively. When cycled again at 100 mA g⁻¹, the discharge capacity of the spinel sample reached 148 mAh g⁻¹ with a capacity retention of 91.5%. Again, rate capability tests show much better performance for the wide-range voltage than the narrow one. 3.6. EIS Measurements Electrochemical impedance spectroscopy is a useful in-situ technique to investigate the changes in the electrode-electrolyte interface resistance and Li⁺ ion kinetics of electrodes (Figure 11). EIS measurements of LMO900 were carried out for the fresh electrode and after the 100th cycle for the voltage window 3.0–4.5. Additionally, measurements were performed after the 1st and 100th cycle for the voltage window 1.5–4.8 V, recorded at a rate of 0.7 C. Figure 11a,b show the Nyquist plots for 1.5–4.8 and 3.0–4.5 V, respectively. The equivalent circuit model used to analyze the EIS patterns (Figure 11e) is composed of four series components: the ohmic resistance of the cell (R_s), the impedance of the SEI layer (R_SEI-CPE_SEI parallel circuit), the charge transfer impedance and interfacial capacitance at the electrode/electrolyte interface (R_ct-CPE_dl parallel circuit), and the Warburg impedance (Z_w-R), which characterizes the Li⁺ ion diffusion-controlled process [67]. In general, there is an increase in the total impedance after the 100th cycle at a 0.7 C rate, as shown from the fitted parameters listed in Table 3. For (1.5–4.8 V), the ohmic resistance (R_s) values are 2.85 and 4.17 Ω after the 1st and 100th cycle, respectively. Also, R_SEI and R_ct significantly increased during cycling. The real part Z’(ω) of the total impedance of the cell is the sum of the real part of the four components: Z_w can be determined from the slope of the Warburg plot Z’ vs. ω^−1/2 (Figure 11c,d), and the apparent diffusion coefficient D_Li is given according to the relation [68]: in which R is the gas constant, T is the absolute temperature, F is the Faraday constant, n is the number of electrons transferred in the redox reaction at the electrode-electrolyte interface, C_Li is the concentration of Li⁺-ion inside the electrode, and A is the effective surface area of the electrode. The apparent diffusion coefficient (D_Li) values for spinel electrodes, both in the initial (fresh) state and after the 1st and 100th cycles, are provided in Table 4. The data is presented for two voltage ranges, namely 3.0–4.5 V and 1.5–4.8 V. In the potential window of 3.0–4.5 V, the apparent diffusion coefficient for the fresh LMO900 electrode is D_Li = 1.26 × 10⁻¹² cm² s⁻¹, which is higher than the value after the 100th cycle (5.9 × 10⁻¹³ cm² s⁻¹). This observation aligns with the increased values of R_SEI and R_ct. As reported previously, the value of D_Li for fresh LMO spinel cathode material varies from 10⁻¹² to 10⁻¹³ cm² s⁻¹ [45,61,69]. By raising the charge potential to 4.8 V and lowering the discharge potential to 1.5 V, the D_Li of 4.53 × 10⁻¹² cm² s⁻¹ after the 1st cycle of the LMO900 electrode is significantly lower than that after the 100th cycle (6.2 × 10⁻¹¹ cm² s⁻¹). We recorded the Nyquist profiles after 1st cycle for the wide voltage window (1.5–4.8 V) because we anticipate a phase transition from cubic LiMn₂O₄ to a tetragonal or spinel-like structure (high Li concentration). This comparison confirms that the expansion of the voltage window (1.5–4.8 V) enhances Li⁺ ion kinetics, possibly attributed to partial spinel-like ordering, Li_1+xMn_2−xO₄, or a partial phase transition to Li₂Mn₂O₄ from the ideal spinel LiMn₂O₄. The deviation from the ideal spinel to a spinel-like or tetragonal structure increases the amount of Li⁺ ions passing through tetrahedral sites or 16c octahedral sites without face-sharing Mn ions. This results in lower electrostatic repulsion compared to when Li⁺ ions pass through tetrahedra that share faces with Mn ions, which leads to improved Li transport kinetics and, thus, a larger capacity. More information on the change in the overall cell potential as a function of the depth-of-discharge (DOD) can be obtained by evaluating the area-specific impedance (ASI) expressed in Ω cm², given by the following relation [70]: where A is the cross-sectional area of the electrode, ΔV = OCV − V_cell is the potential change during current interruption for 60 s at each DOD value, and I is the current passed throughout the cell. Various factors can affect the area-specific impedance, including ohmic drop, Li-ion transport through the electrolyte, and solid-state diffusion within the electrode. Moreover, the ASI does not need to reach equilibrium conditions as in the case of EIS, which makes this technique more representative of the total internal resistance evaluation during cycling. Figure 12 displays the variation of ASI of the LMO900 electrode for the 1st and 100th cycles at 0.7 C rate in the narrow and extended voltage windows. At 20% DOD, the ASI values at the 1st cycle are 1.5 and 6 Ω and increased to 2.5 and 9.7 Ω, after the 100th cycle for the narrow and extended voltage window, respectively. At 90% DOD, the ASI value at the 1st cycle (5.7 Ω) increased to 17 Ω after the 100th cycle for the narrow voltage window (3.0–4.5 V). At the same DOD (90%), the ASI values at the 1st cycle (43.4 Ω) decreased to 39.4 Ω after the 100th cycle for the extended voltage window (1.5–4.8 V). These results indicate that the charge-transfer resistance is dependent on DOD as well as the aging of the electrode. This resistance increases systematically with increasing DOD, as noticed from the narrow voltage window 3.0–4.5 V. For the extended voltage range of 1.5–4.8 V, upon closer examination, as depicted in Figure 12, particularly in the 30–40% depth of discharge (DOD) range, a noteworthy shift (inversion) in the ASI behavior is observed at approximately 32% DOD. This entails a reduction in the ASI values with increased DOD, resulting in decreased charge-transfer resistance. This phenomenon facilitates smoother ion and electron transfer during the charge-discharge process. Comparing the discharge voltages, we observe a consistent increase in ASI values between the narrow and wide voltage windows until the discharge voltage reaches approximately 3 V, corresponding to 32% DOD. At this juncture, it is noteworthy that the voltage value for the wide voltage window is approximately 90% of the discharge voltage range observed in the narrow voltage window, as shown in Figure 12. Therefore, we conclude that the deep discharge at a potential below 3 V may cause a change in the crystal structure of the cathode and thus a change in the behavior of the ASI. This reduction in ASI values, and hence the increase of Li-ion diffusion, may be attributed to a partial spinel-like order, Li_1+yMn_2−yO₄, and a partial phase transition Li₂Mn₂O₄ from ideal spinel LiMn₂O₄. 3.7. Long-term Cycling Characterization To characterize the electrochemical performance of the cathode materials, differential capacity (dQ/dV vs. V) plots are important indicators for tracking phase retention during cycling [34]. The curves dQ/dV vs. V presented in Figure 13 were calculated from GCD data reported in Figure 10b. The peaks at ~4 and ~3 V in the dQ/dV plots correspond to Mn^3.5+/4+ and Mn^3+/3.5+, respectively. In the high potential region, two pairs of redox peaks correspond to the two undistinguished plateaus of Figure 10b, indicating that lithium-ions were extracted and inserted into the spinel phase by a two-step process. The differential capacity plot at the charge stage had two sharp peaks at 4.03 and 4.16 V, and the discharge stage also showed two sharp peaks at 4.01 and 3.97 V. The peak potential at 2.47 V corresponds to the pseudoplateau arising from the insertion of Li ions into the spinel 16c sites. Regarding the differential capacity after 500 cycles, all redox peaks maintain the same potential position; only their intensity decreases throughout cycling due to the capacity decay of the LMO electrode. The peak potential difference remains unchanged after 500 cycles, indicating the good electrochemical stability of this Li-rich electrode, in which doping mitigates the fading mechanisms. Therefore, the differential capacity analysis clearly shows that introducing excess Li in LiMn₂O₄ significantly decreases the rate of capacity degradation during charge-discharge cycling.