Download PDF
Cite This Article
Contents
1. Introduction
2. Materials and Methods
3. Results
4. Discussion
5. Conclusions
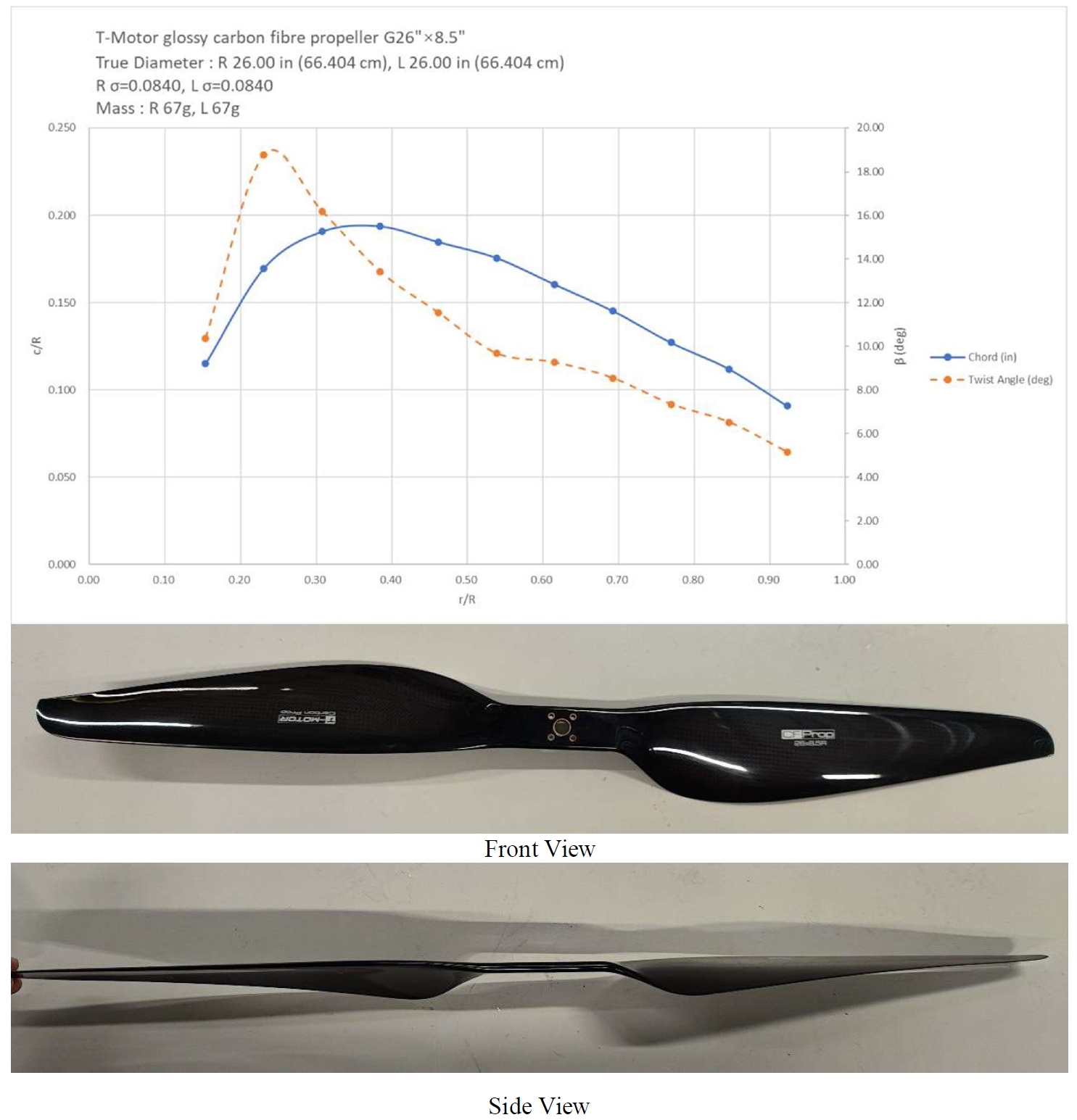
Appendix A. G26″ × 8.5″ Propeller Data
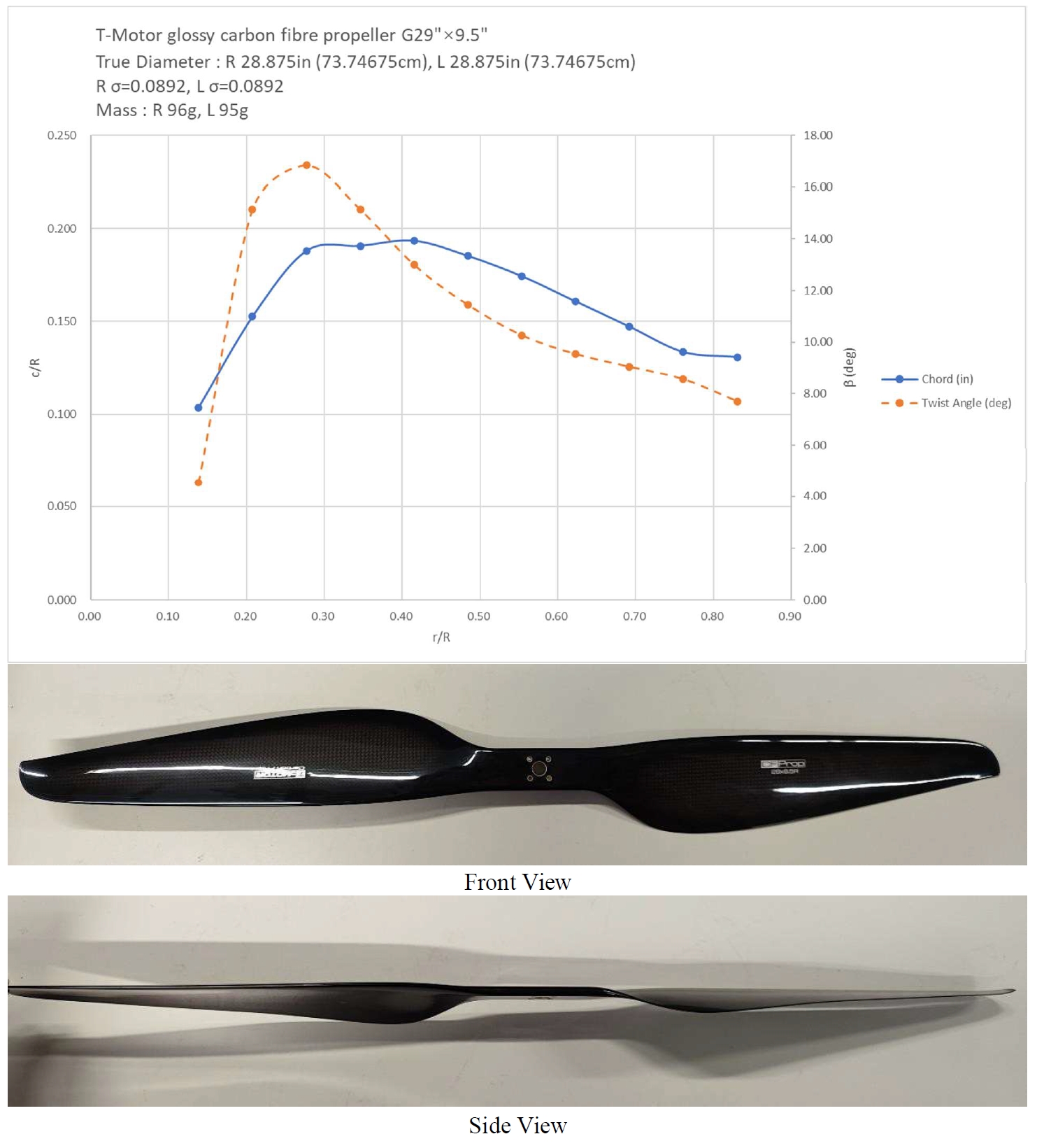
Appendix B. G29″ × 9.5″ Propeller Data
Acknowledgments
Author Contributions
Ethics Statement
Informed Consent Statement
Data Availibility Statement
Funding
Declaration of Competing Interest
References
Investigation of the Performance Characteristics of Unequal Co-Axial Rotors
Author Information
Other Information
Uncrewed Air Vehicles, Aeronautics, Astronautics and Computational Engineering, Boldrewood Innovation Campus, The University of Southampton, Southampton SO16 7QF, UK
*
Authors to whom correspondence should be addressed.
Received: 26 November 2024 Accepted: 02 January 2025 Published: 10 January 2025
© 2025 The authors. This is an open access article under the Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0/).
Drones Veh. Auton.
2025,
2(1), 10002;
DOI: 10.70322/dav.2025.10002
ABSTRACT:
The behaviour of
co-axial rotors is well understood, and they are especially practical for large
UAVs due to their increased thrust without changing the vehicle footprint.
However, for co-axial systems with varying propeller diameters between the two
disks, research is more limited. The goal of this paper was to determine an
optimal configuration for several different unequal co-axial setups using
numerous different propeller combinations and separation ratios. Propellers
with diameters of 26 and 29 inches are tested at separation ratios of 0.05 to
0.35. Thrust and power were collected using an off-the-shelf FS15-TYTO thrust
stand, with the upstream and downstream propellers running at equal throttles.
From this, performance was assessed through efficiency, thrust, and power
consumption, and comparisons were made to an ideal combination without losses.
The results show that for unequal combinations, the user should place the
smaller propeller upstream for greater efficiency, but for maximum thrust
capacity, two equal propellers are preferred. When compared to two independent
rotors of the same size, a 26″ upstream rotor and a 29″ downstream rotor
minimised thrust loss to 16%, compared to 23% for the opposite arrangement. It
was also found that the optimal separation ratio is always approximately 0.2.
Keywords:
Unequal; Propellers; Co-axial; Aerodynamics;
Efficiency; z/D ratio; Optimisation
1. Introduction
Development of co-axial rotor systems has found a resurgence in modern aerospace, largely due to its convenience in increasing the thrust of multirotor Uncrewed Aerial Vehicles (UAVs) whilst keeping a relatively small footprint, something that is especially important for heavy-lift UAVs. This does come at a cost, however, as the complex flow field in the wake of the upstream propeller reduces the performance of the downstream propeller in hover [1,2]. This is unavoidable, although there are ways to mitigate its negative effects. The most common method is by changing the separation ratio (z/D) of the system, which is the ratio of the distance between the blades, z, and the blade diameter, D. Different researchers determine different ideal ratios, but they fall in the general range of 0.1 to 0.4 [3,4]. The result of this is that the downstream (lower) propeller can have a reduction in thrust of 15% to 30% when compared to two independent propellers of the same diameter [5]. There is also a small reduction in the performance of the upstream propeller, although this is much less pronounced [6].
Research into unequal propellers is currently very limited, but the consensus is that a smaller propeller upstream of a larger downstream propeller is the optimal configuration. Ostar-Exel [7] found this to be true for smaller propellers (with diameters from 10 to 15 inches) whilst also confirming that decreasing diameter propellers perform poorly.
These results should be taken with some caution however, as the overall thrust of these unequal systems is expected to be different due to the different disk area in comparison to equally sized systems. Simões [8] also found similar results but for smaller differences in propeller diameter (8 to 17 per cent). Their experiments also concluded that the optimal configuration was to have a smaller upstream propeller than the downstream propeller, although the optimal difference in size between the two propellers was not discussed. A more recent study into the aeroacoustics of co-axial propellers reached similar conclusions, finding that reducing the diameter of the upstream propeller reduces the overall efficiency by 30%, in comparison to only 3% for a likewise change on the downstream propeller [9].
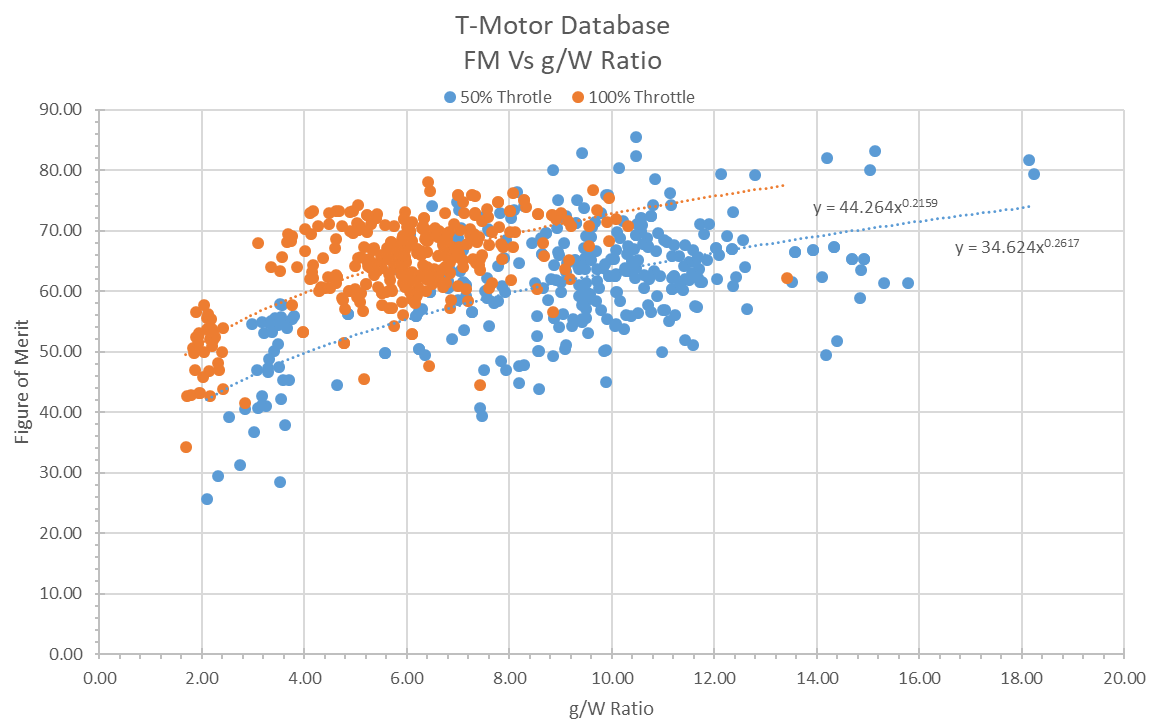
It is intuitive that increasing the angular speed of a propeller will increase the power and thrust, however, this relationship is quadratic for thrust and cubic for power [10]. For co-axial systems, it is important to consider the fact that different propellers can rotate at different speeds. Sunda et al. [11] found that the maximum thrust-torque ratio for a co-axial system in hover was when the upstream propeller and downstream propeller were rotating at the same angular rate. Due to the losses on the downstream (lower) propeller, the upstream propeller is more significant in terms of its impact on the thrust produced. For a given increase in angular velocity, the increase in thrust will be greater if it is the upstream propeller which is rotating faster [12]. This paper also recommends that the two blades are kept at the same rotational velocity. However, they argue this from a mechanical standpoint rather than an aerodynamic standpoint; the torque cancellation provided by operating the propellers this way is beneficial for helicopters, but less significant for multirotor UAVs, where torque cancelling is usually provided by the other rotors on the aircraft. Shuanghou Deng et al. [13] wrote that the thrust, inlet, and exit velocities all increase with an increase in rotational velocity. However, they found that although thrust increases with an increasing velocity, the power loading, and hence efficiency, decreases. Despite this, it is more aerodynamically efficient to have a large, slow moving propeller, rather than a small propeller rotating quickly [14], as lift per unit power (g/W) is proportional to the inverse of the exit velocity of the flow moving through the propeller (see Figure 1).
Figure 1. Graph of the Figure of Merit <i>vs.</i> g/W ratio for 50% & 100% throttle cases, derived from the T-Motor database of 585 propellers.
2. Materials and Methods
2.1. Performance Characteristics
A number of different non-dimensional values can be used to determine the performance of propellers. The ones used in this study are thrust and power coefficients (CT and CP) and Figure of Merit (FM). The definitions of the coefficients are defined below, as found in Leishman’s 2008 paper [15]:
Fundamentally, FM is a ratio of the ideal power required to hover to the actual power required. The definition of FM is more complex for co-axial rotors, but the historical definition for isolated propellers is as follows [16]:
This is a problematic definition, however, as it does not include the fact that each rotor in a co-axial system operates at a slightly different disk loading, as the overall thrust of the system is shared across both propellers. Leishman describes a number of different alternatives that can be used to improve the accuracy of the FM, where each derivation relies on different assumptions. The first alternative definition assumes each rotor shares half the total thrust of the system, W/2 = Tu = Tl and this definition becomes:
Note that CPmeas is the measured system power.
This assumption states that each rotor generates an equal amount of thrust, which is optimistic for co-axial propellers, as it ignores all the aerodynamic losses; for unequal propellers the assumption is even more inaccurate, where the difference in propeller diameter makes it invalid to assume equal thrust sharing. The other cases assume the propellers corotate in the same plane, an idealised assumption that is also not applicable, especially at small separation ratios where aerodynamic interference is most severe. The final option is the definition chosen to be used in this paper, where there are only induced losses, both rotors are in torque balance, and the downstream rotor is in the vena contracta (fully developed slipstream) of the upstream rotor. Using these assumptions, the definition below can be derived, and this is the form that will be used going forward in this paper:
Leishman & Syal derived this equation mathematically using the momentum theory, five possible conditions which would have individual interference effects depending on the arrangement of the rotors (see Table 1). As can be seen in Equation (5) above, Case 4b is the one used in this analysis, i.e., kint = 1.2657. In Leishman & Ananthan’s earlier paper [17], they derived a fourth case whereby the two rotors have a balanced torque. They then derived the interference-induced power factor, kint to be reduced to 1.219, slightly less than the above results.
2.2. Experimental Equipment
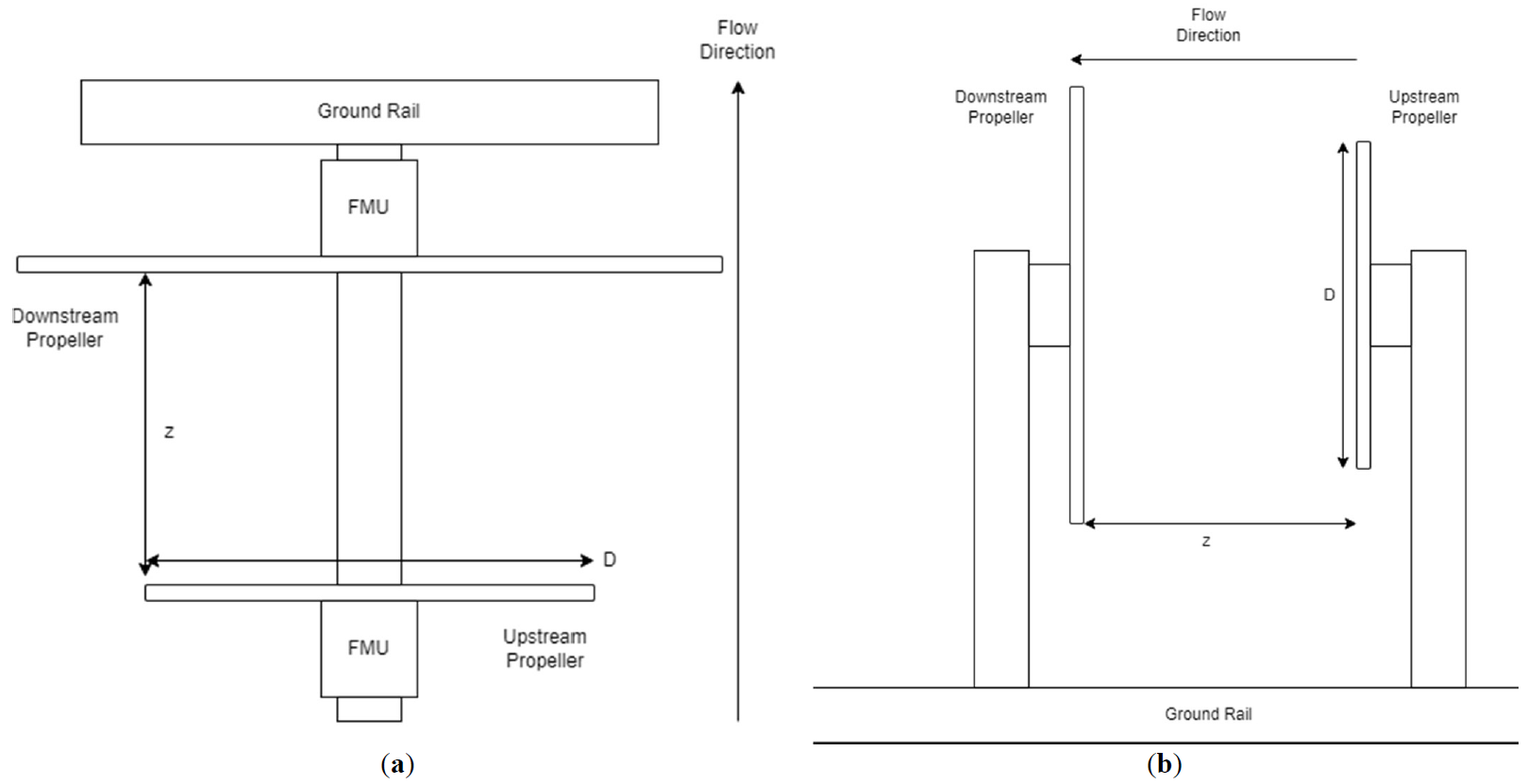
The TYTO Robotics (formerly RCBenchmark, Gatineau, Quebec, Canada) FS15 flight stand was used to measure the data from these propellers. Each propeller was powered by a U8 Kv100 electric motor from T-Motor, although after validating the performance of the motors, its parameters were corrected to Kv80; this change does not influence the validity of the results collected. Each motor was powered by a 25C 6S (22.2 V) Li-Po battery that was fully charged before each test commenced, whilst the motors were each driven by a T-Motor Alpha 60A 6S ESC (Electronic Speed Controller) (Tiger Motor, Nanchang, Jiangxi, China). To create the co-axial setup, the propellers were mounted face-to-face, as can be seen in Figure 2. All propellers had a similar solidity and pitch to diameter ratio, and the properties of each of the tested propellers can be seen below in Table 2.
The uprights, upon which the propellers and Force Measurement Units (FMUs) were mounted, could be moved along the Aluminium extrusions to change the z/D ratio. The minimum limit of 0.05 was as close as the propellers could get before the risk of contact between the blades was too high. The maximum z/D ratio of 0.35 was as far as the uprights could be separated. Operators were protected by securely fastening the rails to the ground and were operating the equipment from behind a protective screen.
2.3. Test Configurations
In this paper, all combinations of 26″ and 29″ T-Motor CF propellers were tested. Each propeller combination was tested at five different z/D ratios, ranging from 0.05 to 0.35, these being 0.05, 0.1, 0.2, 0.3, and 0.35. There is an ambiguity in defining the z/D ratio for unequal propellers, as the ratio can be altered by changing which propeller the ratio is calculated from. This can result in a single separation distance having two slightly different, but equally valid separation ratios. The two ratios are equal when the diameters of the propellers are the same. As the unequal propellers in this study only vary in diameter by around 10%, the difference between the two possible ratios is small. In this paper, the upstream propeller is chosen as the propeller from which the z/D ratio is defined.
2.4. Experimental Procedure
The way in which the data was collected for each test was consistent. A number of different metrics for each propeller were recorded by the thrust stand software, and the ones used in this study are listed below in Table 3. Both propellers in the experiment were run at equivalent throttles; this does not result in balanced thrust or torque between each blade, but this is a more representative operating condition on a UAV, where torque imbalances can be counteracted by the rotor on the opposite corner of the aircraft. This also removes an element of human error in manually setting the throttles to balance torques. Starting at 20% throttle, the system was given time to settle before increasing the throttle by five per cent, up to 90% throttle. A 90% limit was chosen due to measurement limits on the stand. This procedure was repeated at each different separation ratio, for each propeller combination. An image of the co-axial setup can be seen in Figure 3.
Isolated propellers were also tested to provide a baseline to which the co-axial propellers could be compared to. These tests were carried out in a similar fashion to the co-axial tests, with the throttle slowly being ramped up to the maximum. The FMU that had been left empty due to the absence of another propeller was removed from the setup to minimise aerodynamic interference (drag).
```latexC_{T} = \frac{T}{\rho A \Omega^{2} R^{2}}```
```latexC_{P} = \frac{P}{\rho A \Omega^{3} R^{3}}```
```latexF M = \frac{{C_{T}}^{\frac{3}{2}}}{\sqrt{2} C_{P}}```
```latexF M = \frac{{C_{W}}^{\frac{3}{2}}}{2 \cdot C_{P m e a s}}```
```latexF M = \frac{1 . 2657 \frac{{C_{T_{l}}}^{\frac{3}{2}}}{\sqrt{2}} \left[\left(\frac{C_{T_{u}}}{C_{T_{l}}}\right)^{\frac{3}{2}} + 1\right]}{C_{P m e a s}} ```
Table 1. Theoretical predictions of interference-induced power factor, κint.
| Case No. | Interference-Induced Power Factor, κint |
|---|---|
| 1—Corotation in the same plane, at equal thrusts. | 1.4142 |
| 2—Corotation in the same plane, at balanced torques. | 1.4142 |
| 3—Equal thrusts with the lower rotor in the slipstream of the upper rotor. | 1.2808 |
| 4a—Balanced torque with the lower rotor in the slipstream of the upper rotor. | 1.2810 |
| 4b—Same thrust sharing ratio as found in the torque balanced case. | 1.2657 |
Figure 2. Diagram of the propeller testing arrangement at the maximum z/D ratio (separation). (<b>a</b>) Plan-view of the equipment. (<b>b</b>) Side-view of the equipment.
Table 2. T-Motor Propeller specifications (see Appendices A and B).
| Diameter (in) | Handedness | Pitch (in) | P/D Ratio | Mass (g) | Solidity |
|---|---|---|---|---|---|
| 29 | R | 9.5 | 0.3276 | 96 | 0.0892 |
| 29 | L | 9.5 | 0.3276 | 95 | 0.0892 |
| 26 | R | 8.5 | 0.3269 | 67 | 0.0840 |
| 26 | L | 8.5 | 0.3269 | 66 | 0.0840 |
Table 3. Data collected from each test.
| Measured Data | Unit | TYTO’s Stated Operational Limits |
|---|---|---|
| Thrust | N | 150 N |
| Current | A | 180 A |
| Torque | Nm | 8 Nm |
| Rotational Speed | rpm | 30,000 rpm |
| Electrical Input Power | W | 27 kW |
| Mechanical Output Power | W | 27 kW |
3. Results
3.1. The Unique Case of Equal Throttle
Running the experiments with the throttles balanced creates an interesting use case, as most theoretical approaches, as well as experimental approaches, use the case of equal thrust, rotational speed, or more commonly, equal torque. For unequal propellers, it becomes harder to use these metrics as the smaller propeller will eventually be unable to match the thrust or torque of its larger counterpart. Due to this nature, it makes it impractical to test in this fashion, as not enough data points can be collected before the smaller propeller is maxed out; there is scope for future research into how big the difference between these scenarios can be before saturation occurs.
3.2. Differences in Individual Propeller Performance
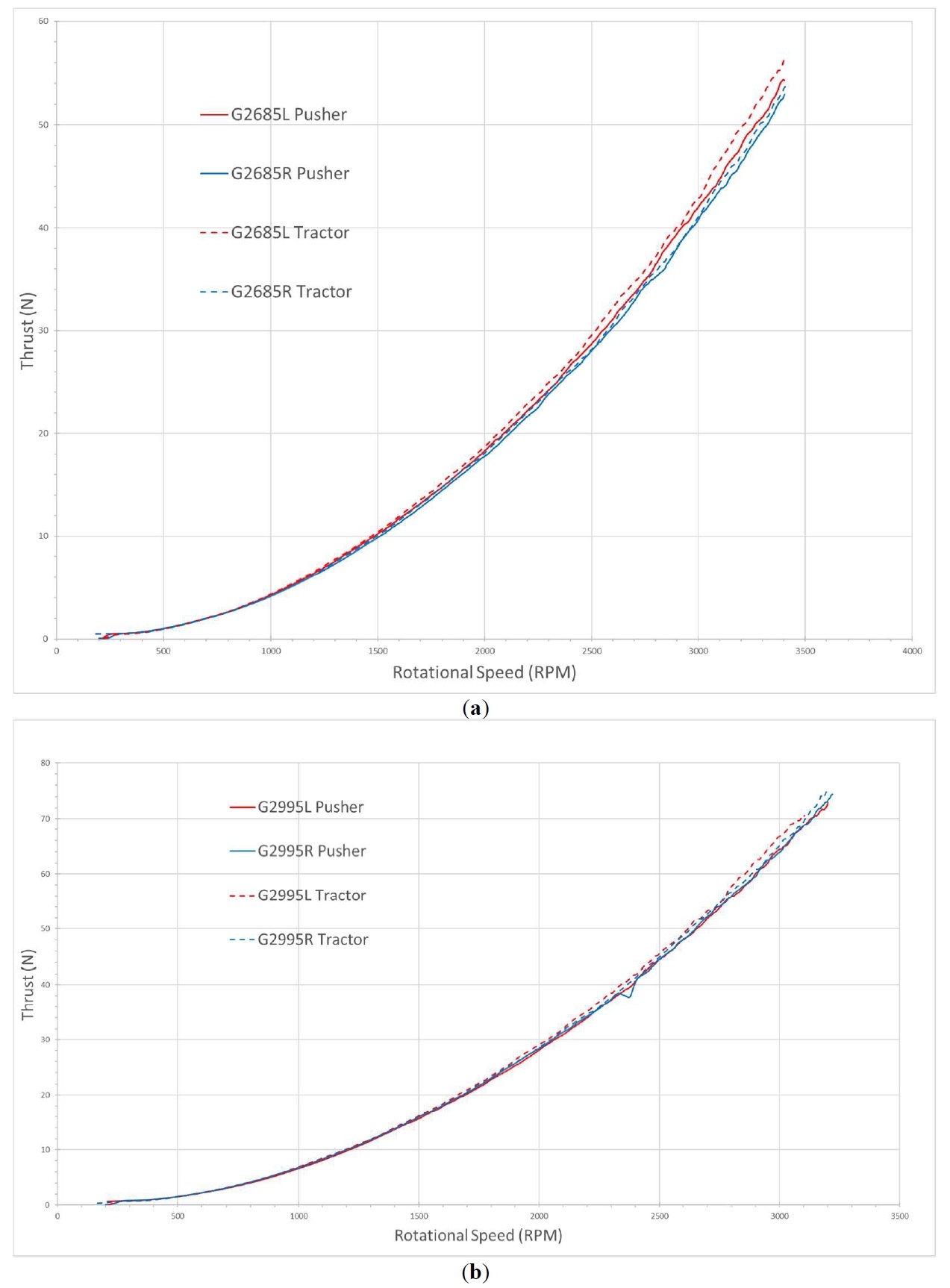
Due to slight manufacturing differences in the propellers, it is possible that the left and right-handed counterparts of the same propeller would perform differently from each other. The right-handed propellers are all slightly heavier by 1–3 g in comparison to their left-handed equivalents. It is also expected that propellers acting as a tractor will generate less thrust (up to 5%) than an otherwise equivalent pusher propeller. This is due to the lack of obstruction in the wake of the propeller affecting the thrust output. However, it can be seen below in Figure 4a,b that the opposite is true. A likely explanation for this phenomenon is that the FMU on the tractor propeller is interfering in two ways: firstly, by measuring the reaction force of some of the airflow hitting its surface, whilst also reflecting some of this back into the prop disk, artificially increasing the measured thrust. These differences do not create a significant difference in performance, only a couple of Newtons at maximum thrust, butnonetheless, the handedness and direction of the propeller will be considered when selecting a reference point for comparing the co-axial propellers.
In order to reduce complication, the results for Sections 3.3–3.7 will reference the tests completed at a z/D ratio of 0.2, as this is the ratio where performance is expected to be best [18]. The variations in performance across the full range of separation ratios will be discussed in Section 3.8.
3.3. Thrust Loss
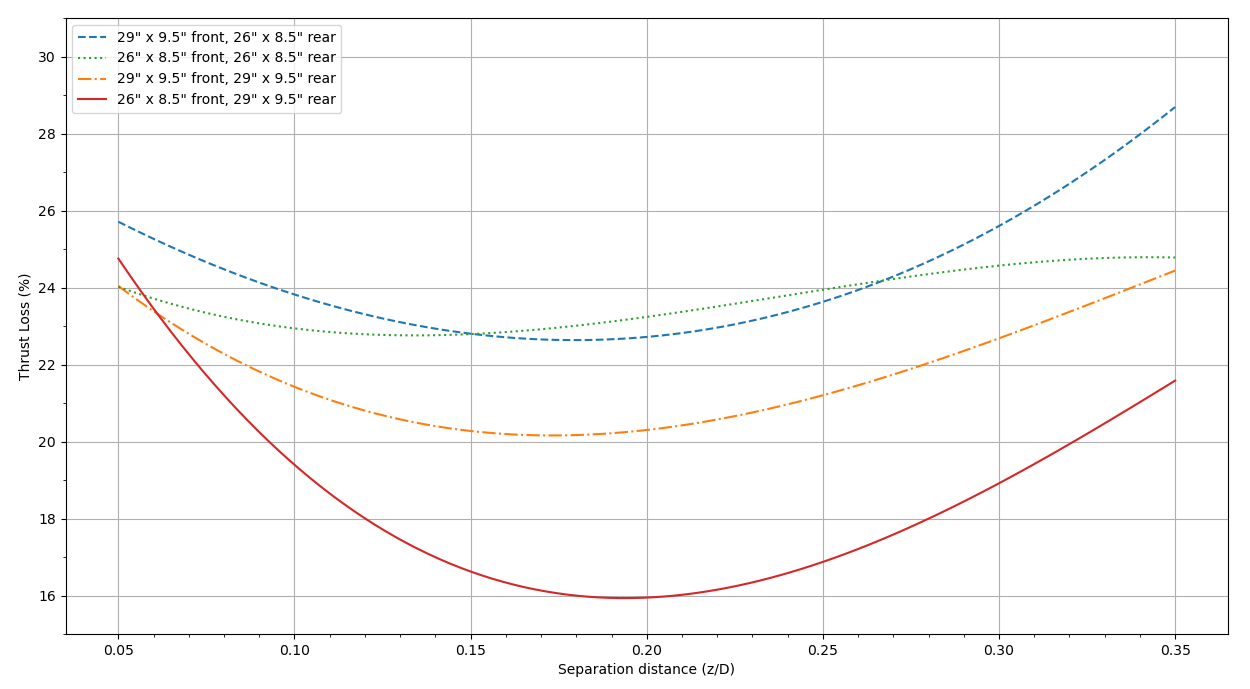
In this section, the thrust of a co-axial system is compared to an ideal co-axial powertrain, i.e., two isolated propellers acting independently of each other, where there is no interference between them. This shows the effect of the wake coming off the upstream propeller in reducing the thrust output of the downstream propeller, and can be used to determine the difference in thrust between the co-axial system and an ideal co-axial pair, i.e., two independent propellers. Figure 5 below clearly shows a significant difference in performance when comparing the different combinations. The ideal combination to minimise the aerodynamic losses is the unequal pair with the smaller propeller leading (upper), in this case, a 26″ upstream propeller leading to a 29″ downstream propeller.
This results in a reduction in the thrust of 17.3%, in contrast to the opposite combination, which has a reduction in thrust of 23.8%. The pair of small propellers suffers a similarly large thrust loss percentage to the poorly performing equal pair. It could be expected that the two equal configurations would have an equal performance, although that is clearly not the case. This is likely caused by the smaller blades operating at a higher rotational speed. As blade efficiency is inversely proportional to airflow exit velocity [14], it makes sense that the lower rotational speed, and hence mass flow rate, of the larger propeller would be more efficient and have a smaller reduction in thrust.
3.4. Power Increase
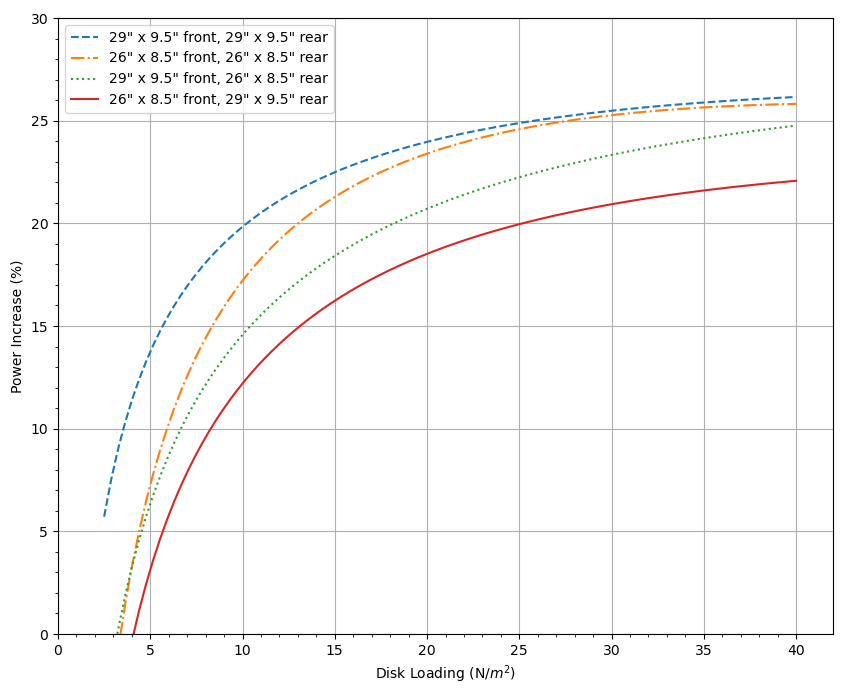
Another side effect of the downstream propeller being in the turbulent wake is that the power required to generate as much thrust as an isolated propeller increases, and consequently, the power consumption of a co-axial system is greater than that of an ideal system. Using the same definition for an ideal propeller as in Section 3.3, a similar plot for the percentage power increase of each co-axial configuration can be generated, and that is below in Figure 6, where trendlines are shown.
Also shown is that the power increase is small at low disk loadings before increasing rapidly and plateauing in a logarithmic profile as disk loading increases. Both unequal pairs see a smaller increase in power at any disk loading in comparison to the equal pairs, with the small-leading pair seeing the smallest increase, at only 22.7%. Both equal pairs have a maximum of around 27%, with the other unequal pair falling at 25.5%. This is a different result in comparison to thrust loss, as in the case of power, even the large-leading unequal pair outperforms both equal pairs. The difference in behaviour could be caused by the smaller rear propeller drawing a smaller fraction of the overall power of the system, so the interference on it has a reduced effect on the system as a whole.
3.5. Total Thrust
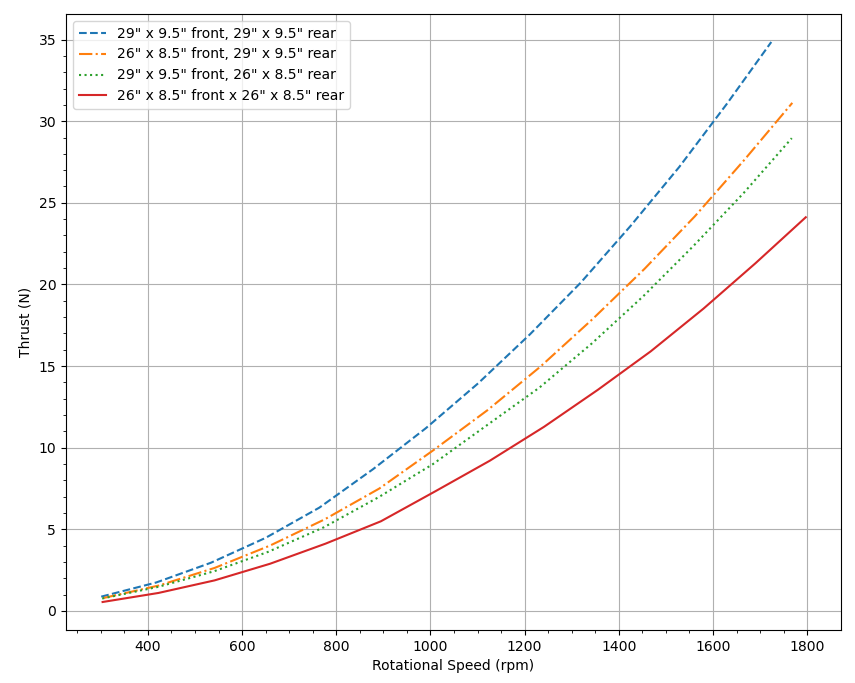
It is also helpful to consider the gross thrust of each system for a final use case; when an aircraft is being designed, it may be preferable to design for maximum thrust capacity rather than for efficiency, and the total thrust produced by the different co-axial pairs is shown in Figure 7. When considering total thrust, the biggest factor that influences it is simply the total disk area, and this is not surprising given the relatively small increases in efficiency described previously. The efficiency of arranging an unequal propeller is clear, with the pair where the smaller propeller is placed upstream producing 2.2 N more thrust at maximum throttle, or a 7.5% increase. However, this is a less significant difference in comparison to the equal co-axial pair with larger propellers; the pair of 29″ propellers produced a maximum thrust of 34.9 N, or 3.7 N (12%) more than the optimal unequal configuration discussed above.
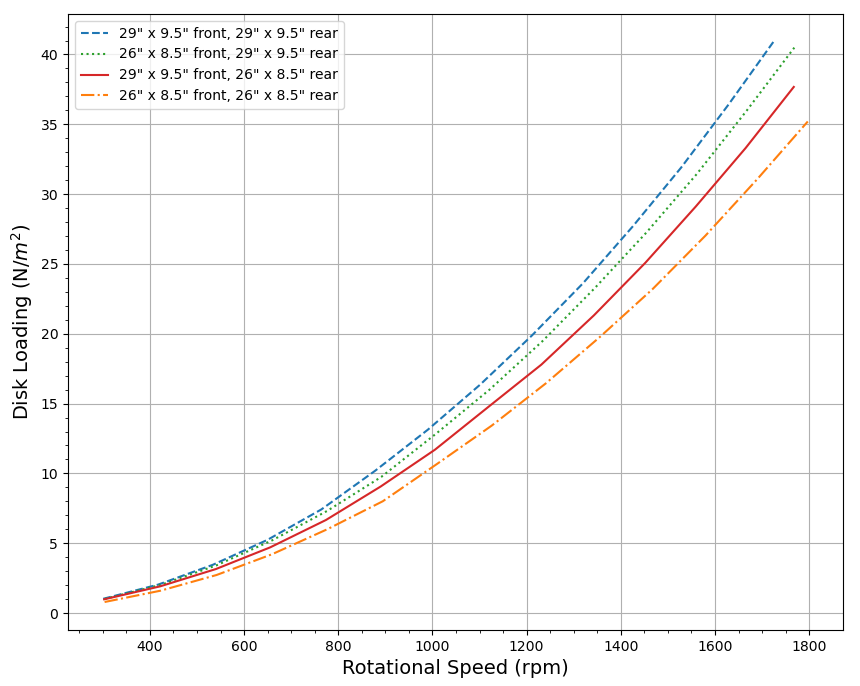
To try and eliminate the propeller size as an influencing factor, the total thrust can be normalised to the disk area of the co-axial propeller, also known as the disk loading, and this is shown in Figure 8. For this case, the total disk area is used, i.e., the sum of the areas of both the upstream and downstream propellers. This operation reduces the deficit between the equal and unequal propellers, but the equal pair with the two larger propellers remains optimal by this definition—the pair of 29″ propellers produces the greatest disk loading at a given rpm, whilst also producing the maximum outright disk loading. As before, the small unequal pairing performs worse in comparison to the larger equal pair, and this is again caused by it running at a reduced efficiency at higher rotational velocities.
3.6. Electrical and Mechanical Power
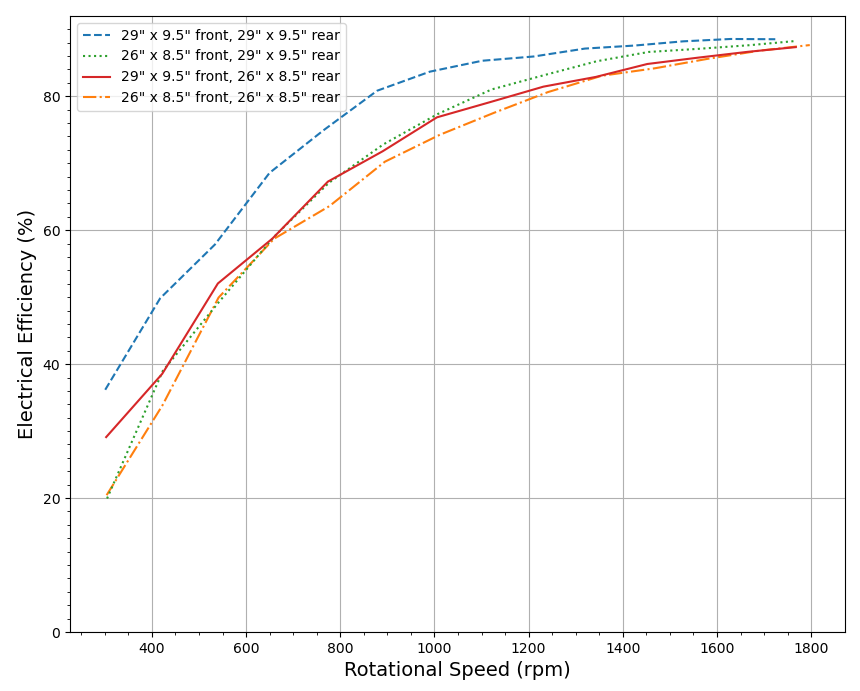
When analysing the performance of a powertrain, it is also important to consider the electrical efficiency. The test stand collects both the input electrical power from the batteries as well as the mechanical power generated by the propellers. The electrical efficiency of the co-axial systems can be seen in Figure 9, and it is calculated as the ratio of output power to input power. The overall trend is that efficiency is very poor at low rpms, before increasing rapidly with rpm, and then plateauing between 0.87 and 0.89. Most of the configurations have approximately the same efficiency, in fact, the difference between the most and least efficient is only 1.4% at maximum rpm. Despite all converging at higher throttles, the pair of large propellers is noticeably more efficient across a large range of rotor rpm, suggesting that the motors are more suited to operating with the larger propellers.
The mechanical power of the different configurations was also analysed, and Figure 10 shows the thrust generated by the powertrain at a given power. It shows that, while not being able to produce the maximum thrust of the large pair, the unequal pair with the small propeller leading is just as effective at producing thrust for a given power, despite the previously mentioned discrepancies created by the motors’ preference for larger props. At 150 W, the aforementioned equal and unequal pairs generate 23.9 N and 23.8 N, respectively, a difference of only 0.4%. The other unequal pair here is also clearly worse than its unequal counterpart, across the whole spectrum, generating 3.7% less thrust at 150 W, whilst also having a much smaller maximum thrust.
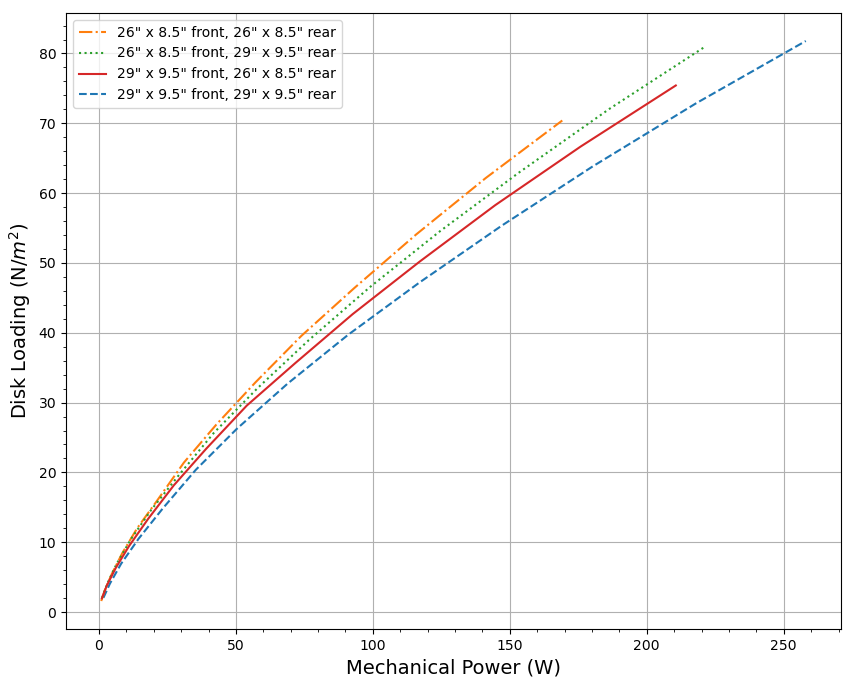
The same technique of normalising the thrust to disk area can be applied here, and is shown in Figure 11. As before, the sum of both propeller areas is used. Again, the optimal unequal configuration is clear, with the upstream small propeller outperforming its unequal counterpart. At 150 W, the small pair has a disk loading of 32.8 N/m2, compared to 31.0 N/m2 for the 26″ by 29″ unequal pair, and 29.8 N/m2 and 28.0 N/m2 for the other unequal and equal pairs, respectively.
3.7. Figure of Merit
As stated in Section 2.1, there are different definitions that can be used when discussing FM, but Equation (5) is the definition used in this section.
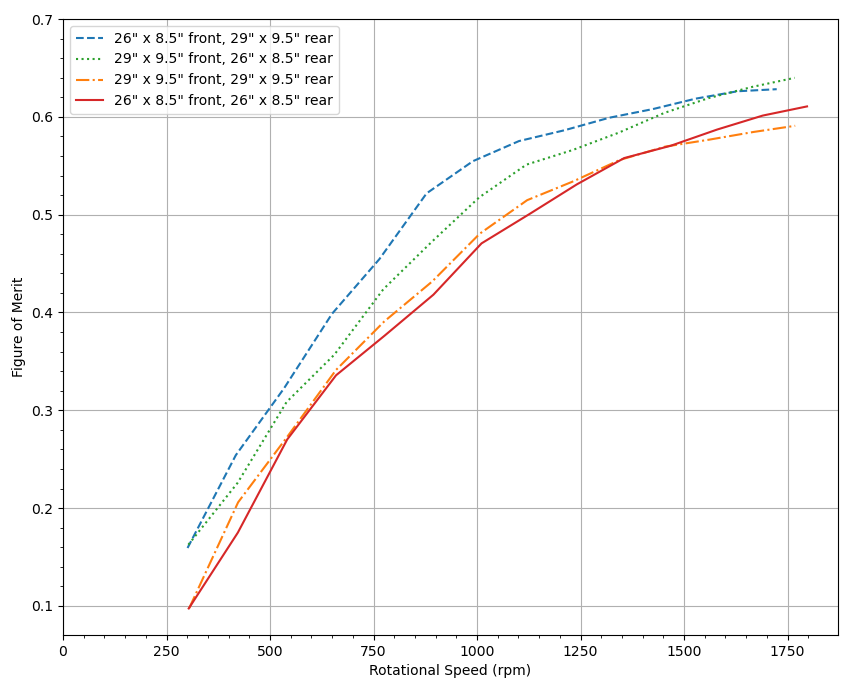
The FM of the various configurations is shown in Figure 12. It is clear that the efficiency of the propellers (rotors) increases significantly as the rotational speed (rpm) increases, similarly to the electrical efficiency, although the plateau is less pronounced. Both equal pairs have similar performance across the whole range of rotational speeds, and both perform worse than the unequal co-axial pairs.
The peak efficiency for the unequal propellers is 62.8% for the small leading and 64.0% for the large leading, a large increase in efficiency in comparison to 59.0% and 61.0% for both the large and small equal propeller pairs. This is surprising, considering all evidence previously suggests that the unequal pair with the large propeller leading should be the least efficient. Generally, across the range of rotational speeds, the other unequal pair is the most efficient, although the difference between the two unequal configurations is small at the maximum throttle values.
3.8. Separation Ratio
It is important to consider how the performance of co-axial rotors changes as the separation ratio is changed. To reiterate what was mentioned in Section 2.3, the separation ratio is ambiguous for co-axial rotors, but in this analysis the diameter of the upstream propeller is used for this analysis. Due to the lack of separation ratios tested, the Figures in this Section are plotted with fitment lines when appropriate, to approximate the behaviour at intermediary separation ratios.
3.8.1. Thrust Loss
Firstly, the thrust loss, as defined in Section 3.3, can be measured across all separation ratios. Starting from a small separation, the performance is expected to be poor before improving as the separation is increased, but after a point, the performance will tail off again. This pattern is clearly visible across all separation ratios, as shown in Figure 13. All the arrangements have the expected ‘bucket’ shape, with sharp decreases in performance at the extreme separations of 0.05 and 0.35. It is expected that the performance degrades at a small separation distance due to the extreme aerodynamic interference between the different propellers. At higher separation ratios, the rear (lower) rotor carries more of the total thrust for the system, and this causes a drop-off in performance as the rear propeller is never as efficient as the front propeller [19].
The small equal pair has a less pronounced shape when compared to the other configurations; the differences between the extremes are less pronounced, and the dip between the extremes is smaller. This suggests that the small propeller is less sensitive to changing the separation distance in comparison to the larger propellers.
The optimum separation distance for performance is in the range of 0.1 to 0.3, but the exact point of peak performance varies slightly between the different pairs: for the unequal pair with the small propeller upstream, the optimal separation ratio is 0.2, but for the other three it is closer to 0.1. This aligns with the theoretical optimum separation distance being 0.2 as mentioned previously. The variation in optimal separation between the equal and unequal propellers could be due to the difference in the way the separation distance is defined in co-axial systems—z/D is defined here off the large propeller in the unequal systems, and these results vary if the propeller chosen is the smaller one. In other words, it may be an artefact of the definition of the z/D ratio as opposed to a physical difference.
3.8.2. Figure of Merit
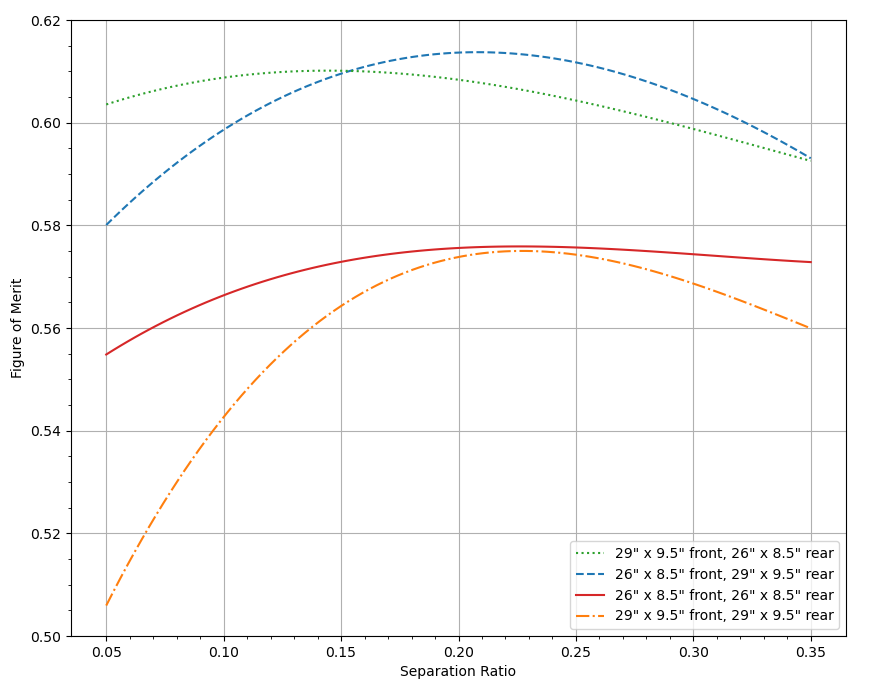
As the thrust loss can be compared across separation ratios, so can the Figure of Merit. In order to determine a single FM value, an average of the high-throttle points was taken. The characteristic shape is also clear, with distinct decreases in performance towards the extreme separation ratios. However, the fall in performance at the extremes is less pronounced here than the thrust loss. The range of FM across all the separation ratios is quite small—the difference between the minimum and maximum across all different combinations is only 0.11, and the trends shown in the FM in Section 3.4 are continued across all separation ratios; those trends being that the equal pairs perform worse than the unequal pairs, and that the ideal configuration is the small propeller leading. Figure 14 shows that this fact is not true across all separation ratios, as the other unequal pair is more efficient at the extreme separation ratios. This could be due to the wake from the front propeller not being fully formed and having a smaller impact.
The ideal separation ratio is not the same for each of the different configurations. For the large-leading unequal pair, the most efficient ratio is 0.1. before slowly decreasing in efficiency as the separation ratio increases. This contrasts with the other arrangements, where the ideal separation is around 0.2, with clear decreases in performance on either side of this mark.
3.8.3. Power Increase
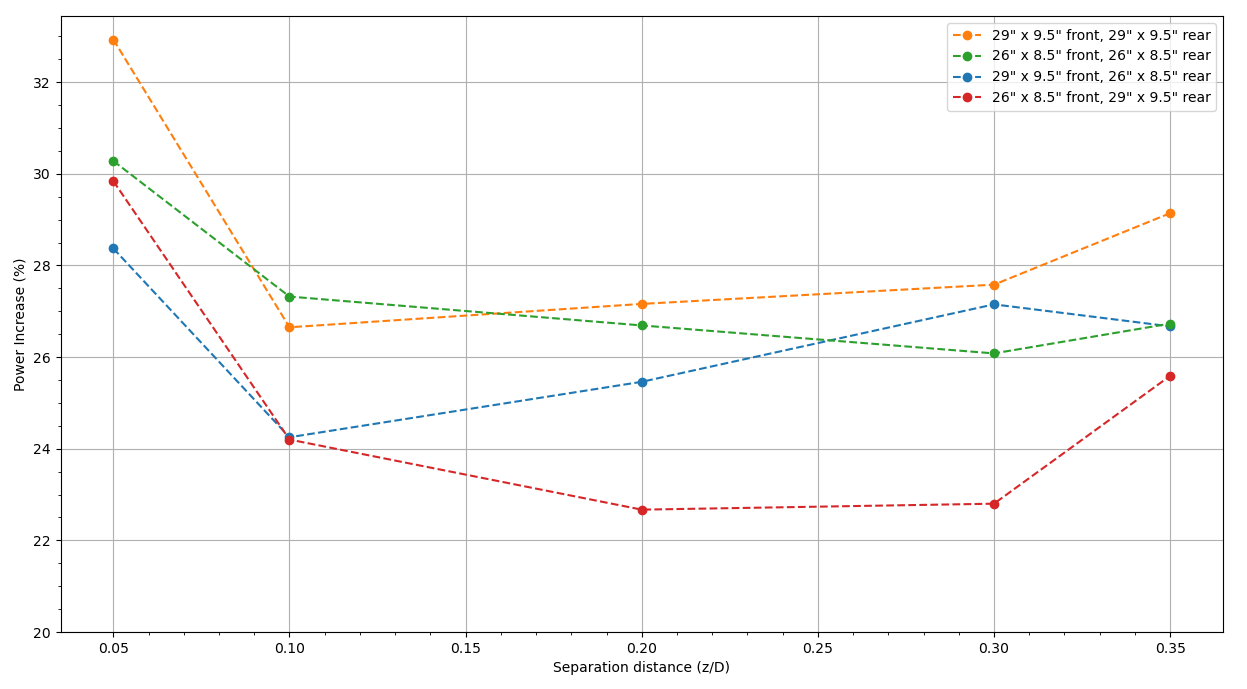
The increase in power of the co-axial pairs follows a similar trend to the other parameters across different separation ratios, and this is shown below in Figure 15. The small-leading unequal pair is clearly the optimum performing configuration, with its minimum increase at z/D = 0.2 being 12 to 18% smaller than all the other configurations. This pair also has the most distinct ‘trough’ profile of all the configurations, but the optimal separation ratio is not obvious as the difference between 0.2 and 0.3 is less than 1%.
Both the large-leading and equal-small pairs show this ‘trough’ profile, although it is less distinct than the small-leading pair. Strangely, the performance of the large-leading pair improves at the maximum separation ratio tested, although this is likely just an anomalous reading. The ideal separation ratio for each pair is different, but hard to determine specifically. It does, however, always fall in the range of 0.1 to 0.3.
Figure 4. The thrust of isolated propellers, comparing handedness and direction (tractor or pusher). (<b>a</b>) Thrust comparison for the 26-inch propeller. (<b>b</b>) Thrust comparison for the 29-inch propeller.
Figure 5. Percentage thrust loss for the co-axial system in comparison to an ideal system, at a z/D ratio of 0.2.
Figure 6. Percentage power increase for the co-axial system in comparison to an ideal system, at a z/D ratio of 0.2.
Figure 7. Total thrust produced by co-axial propellers at a z/D separation ratio of 0.2.
Figure 8. Co-axial disk loading with reference to rpm at a z/D separation ratio of 0.2.
Figure 9. BLDC Motor Electrical Efficiency across different co-axial configurations at a z/D separation ratio of 0.2.
Figure 10. Total Thrust-Power relationship for co-axial pairs at a z/D separation ratio of 0.2.
Figure 11. Disk loading with respect to power for the co-axial pairs, at a z/D separation ratio of 0.2.
Figure 12. Figure of Merit (using the new definition) for the co-axial propellers at a separation ratio z/D of 0.2.
Figure 13. Thrust losses for equal and unequal co-axial powertrains, when compared to an ideal co-axial pair (two independent rotors) with no losses.
Figure 14. Variation of Figure of Merit with z/D separation ratio.
Figure 15. Power increase percentage across z/D separation ratios.
4. Discussion
It is noted that the ‘best’ configuration changes depending on how the performance of the configurations are quantified. For example, when measuring for efficiency by using the Figure of Merit, it appears that the ideal setup is an unequal pair, with a smaller propeller upstream. This is different to if an ideal setup is one with the maximum thrust, as in this case maximising disk area will create the best conditions. These choices, namely maximum thrust or efficiency, determine the conclusions drawn in all cases, and whether adjusting for total disk area, using electrical or mechanical power, or by using different definitions for FM, all lead the conversation on performance in different directions.
Across different separation ratios, there are distinct differences in performance for all rotor combinations. The exact optimal separation ratio for each separation ratio cannot be determined from this study, and the reasons are twofold. Firstly, the ideal ratio changes depending on the performance metric being used. Secondly, not enough distinct ratios were tested to select an exact point. There are, however, clear indications that ideal performance falls within the range of 0.1 to 0.3, with thrust loss being minimised at smaller separations, and FM being maximised at higher separations. The sharp drop-off in performance at the extremes is to be expected, as at small ratios, the aerodynamic interference between the two rotors is the most extreme, and at high ratios, the inefficient downstream rotor takes up a larger fraction of the total thrust.
There are limitations in the collection of data in this study. It is unavoidable that the measurement apparatus will interfere in the wake of the propellers, and this has a small but measurable impact on the results. Also due to the nature of the electric motors used in this experiment, there is a natural bias in performance towards larger propellers, and unfortunately, it is impossible to use a motor that is equally efficient for different propeller diameters. Small changes introduced in the manufacturing process led to changes in mass and profile across theoretically identical propellers, although this was measured and accounted for where possible. In addition, the different propellers all have a different pitch. Although the pitch ratios are similar across the diameters, the differences, especially in the downstream propeller where swirl recovery is important and dependent on the pitch [20], will have an impact on the performance.
5. Conclusions
The objective of this study was to analyse how varying the diameter of the propellers in a co-axial system affects performance, through measuring efficiency and losses, and by comparing it to ideal scenarios. Unequal propellers show similar patterns of performance to equal propellers across a range of separation ratios, with the optimal ratio being somewhere in the range between 0.1 and 0.3, and this is true no matter the setup of the propellers. More separation ratios need to be tested in order to more accurately specify the optimal ratio.
When running unequal propellers, it is optimal to have the upstream propeller be a smaller diameter than the downstream propeller. This arrangement results in an increase in efficiency and a reduction in power required when compared to an oppositely arranged unequal pair, and this manifests itself as a reduction in the thrust loss on the rear propeller from 23% to 16%. Despite this, to generate the most thrust, it may be best to sacrifice the efficiency gained from using unequal rotors in order to increase the total disk area; the maximum thrust produced is from the two largest propellers, and this is 12% more than the most efficient unequal combination.
It is not possible to empirically say which configuration is best, as it is important to consider other factors outside of just aerodynamic performance, such as mass, size, and/or cost. For example, the small-large propellers do not produce as much thrust as the large-large pairs, but they will be lighter and cheaper. Therefore, different configurations are suitable for different applications: if it is desired to add more thrust for the minimum weight, then the small-large pair is best, but if the main consideration is gross thrust, then a large equal co-axial pair will be a better option.
Appendix A. G26″ × 8.5″ Propeller Data
Appendix B. G29″ × 9.5″ Propeller Data
Acknowledgments
We hereby acknowledge the administrative and technical support from within the University of Southampton, which enabled this work to be undertaken.
Author Contributions
This research article was written by both authors, the individual contributions are as follows: Conceptualization, S.D.P. and M.M.; methodology, S.D.P. and M.M.; software, M.M.; validation, S.D.P. and M.M.; formal analysis, M.M.; investigation, S.D.P. and M.M.; resources, S.D.P.; data curation, S.D.P. and M.M.; writing—original draft preparation, M.M. and S.D.P.; writing—review and editing, S.D.P. and M.M.; visualization, M.M. and S.D.P.; supervision, S.D.P.; project administration, S.D.P.; funding acquisition, S.D.P. All authors have read and agreed to the published version of the manuscript.
Ethics Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availibility Statement
A range of propeller test data is available online via the UIUC Propeller Data Site, Department of Aerospace Engineering, Vol. 1–4. This can be accessed for free from: https://m-selig.ae.illinois.edu/props/propDB.html (accessed on 11 November 2024).
Funding
This research was funded by the Engineering and Physical Sciences Research Council (EPSRC), grant number (EP/V05614X/1), entitled “Aerodynamics and aeroacoustics of closely coupled rotors”. This research funding also paid for the APC charges.
Declaration of Competing Interest
The authors declare no conflicts of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript; or in the decision to publish the results.
Nomenclature
References
1.
Chaitanya PJP, Prior SD, Parry AB. On the optimum separation distance for minimum noise of contra-rotating rotors. J. Sound Vib. 2022, 535, 117032. [Google Scholar]
2.
Xu ZY. Numerical Simulation of Unsteady Flow Around Forward Flight Helicopter with Coaxial Rotors. Chin. J. Aeronaut. 2011, 24, 1–10. [Google Scholar]
3.
Shukla NKD. Drone Scale Coaxial Rotor Aerodynamic Interactions Investigation. J. Fluids Eng. 2019, 141, 071201. [Google Scholar]
4.
Bell MB, Prior SD, Erbil MA, Karamanoglu M. Development of a Test-Rig for Exploring Optimal Conditions of Small Unmanned Aerial Vehicle Co-Axial Rotor Systems. In Proceedings of the International Conference on Manufacturing Engineering Systems, Taiwan, Republic of China (ROC), 16–20 December 2010.
5.
Zhu SN, He YL, Chen CX, Wang SY. Research on Hover Characteristics of Ducted Fan with Coaxial Rotors. Appl. Mech. Mater. 2013, 427, 216–221. [Google Scholar]
6.
Lim KWMJ, Johnson W. Hover Performance Correlation for Full-Scale and Model-Scale Coaxial Rotors. J. Am. Helicopter Soc. 2009, 54, 032005. [Google Scholar]
7.
Ostar-Exel L. The Effects of Varying Diameter on Coaxial Propellers for the Propulsion of Multirotor Systems. PhD Thesis, School of Graduate Studies, The State University of New Jersey, Brunswick, NJ, USA, 2019.
8.
Simões M. Optimizing a Coaxial Propulsion System to a Quadcopter Cédric. Master’s Thesis, Department of Engenharia Mecânica, Instituto Superior Técnico, Av. Rovisco Pais, Lisboa, Portugal, 2014.
9.
Palleja-Cabre SCP, Joseph P. Acoustic and Aerodynamic Performance of Co-Axial Contra-Rotating Rotors with Unequal Diameters. In Proceedings of the 30th AIAA/CEAS Aeroacoustics Conference, Rome, Italy, 4–7 June 2024.
10.
Cai GM, Li ZX. Aerodynamic Characteristics of a Ducted Fan System Based on Momentum Source Method. In Proceedings of the 3rd International Conference on Fluid Mechanics and Industrial Applications, Taiyun, China, 29–30 June 2019.
11.
Sunada KTS, Kawashima K. Maximization of Thrust-Torque Ratio of a Coaxial Rotor. J. Aircraft 2005, 42, 300–307. [Google Scholar]
12.
Che Muhammad Ikram CF, Che Umar M. Distance and Rotational Speed Analysis of Coaxial Rotors for UTHM CDrone. Prog. Aerosp. Aviat. Technol. 2021, 1, 45–55. [Google Scholar]
13.
Deng SW, Zhang Z. Aerodynamic Performance Assessment of a Ducted Fan UAV for VTOL Applications. Aerosp. Sci. Technol. 2020, 103, 105895. [Google Scholar]
14.
Fahlstrom TJG. Introduction to UAV Systems, 4th ed.; Wiley: Hoboken, NJ, USA, 2012; p. 76.
15.
Leishman GSJ. Figure of Merit Definition for Coaxial Rotors. J. Am. Helicopter Soc. 2008, 53, 290–300. [Google Scholar]
16.
Coleman CP. A Survey of Theoretical and Experimental Coaxial Rotor Aerodynamic Research. Available online: https://ntrs.nasa.gov/citations/19970015550 (Accessed on 1 November 2024).
17.
Leishman JG. Aerodynamic Optimization of a Coaxial Proprotor. In Proceedings of the American Helicopter Society 62nd Annual Forum, Phoenix, USA, 9–11 May 2006.
18.
Prior SD. Reviewing and Investigating the Use of Co-Axial Rotor Systems in Small UAVs. Int. J. Micro Air Veh. 2010, 2, 1–12. [Google Scholar]
19.
Fernandes SD. Performance Analysis of a Coaxial Helicopter in Hover and Forward Flight. Master’s Thesis, Embry-Riddle Aeronautical University, Prescott, AZ, USA, 2017.
20.
Holzsager JE. The Effects of Coaxial Propellers for the Propulsion of Multirotor Systems. PhD Thesis, School of Graduate Studies, Rutgers University, New Brunswick, NJ, USA, 2017.